Atiyah-Floer conjecture
A conjecture relating the instanton Floer homology of suitable three-dimensional manifolds with the symplectic Floer homology of moduli spaces of flat connections over surfaces, and hence with the quantum cohomology of such moduli spaces. It was originally stated by M.F. Atiyah for homology  -spheres in [a1]. The extension of the conjecture to the case of mapping cylinders was prompted by A. Floer and solved in this case by S. Dostoglou and D. Salamon in [a3].
-spheres in [a1]. The extension of the conjecture to the case of mapping cylinders was prompted by A. Floer and solved in this case by S. Dostoglou and D. Salamon in [a3].
Instanton Floer homology for three-dimensional manifolds was introduced by Floer in [a10]. Let 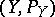 be a pair consisting of a closed oriented
be a pair consisting of a closed oriented  -dimensional manifold
-dimensional manifold 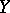 and an
and an 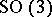 -bundle
-bundle 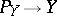 . If either
. If either 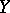 is a homology
is a homology  -sphere or
-sphere or  and the second Stiefel–Whitney class
and the second Stiefel–Whitney class 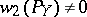 , then the instanton Floer homology
, then the instanton Floer homology 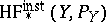 is defined as the homology of the Morse-type complex constructed out of the Chern–Simons functional. The critical points are flat connections and the connecting orbits are anti-self-dual connections on
is defined as the homology of the Morse-type complex constructed out of the Chern–Simons functional. The critical points are flat connections and the connecting orbits are anti-self-dual connections on 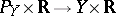 decaying exponentially to flat connections
decaying exponentially to flat connections  when
when 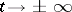 .
.
The symplectic Floer homology for Lagrangian intersections was introduced by Floer in [a11]. Let  be a symplectic manifold which is monotone and simply connected. Let
be a symplectic manifold which is monotone and simply connected. Let 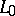 and
and 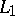 be Lagrangian submanifolds of
be Lagrangian submanifolds of 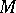 . Then there are Floer homology groups
. Then there are Floer homology groups 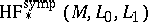 . Now the critical points are the intersection points
. Now the critical points are the intersection points  and the connecting orbits are
and the connecting orbits are  -holomorphic strips
-holomorphic strips 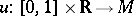 with
with 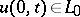 ,
, 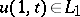 and
and 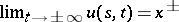 , where
, where 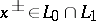 and
and  is an almost-complex structure compatible with the symplectic form.
is an almost-complex structure compatible with the symplectic form.
Let 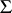 be a closed oriented surface of genus
be a closed oriented surface of genus  and let
and let 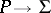 be the trivial
be the trivial 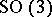 -bundle. Then the moduli space
-bundle. Then the moduli space 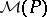 of flat connections on
of flat connections on  is symplectic and smooth except at the trivial connection. Now, let
is symplectic and smooth except at the trivial connection. Now, let 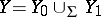 be a Heegaard splitting of a homology
be a Heegaard splitting of a homology  -sphere and consider the trivial
-sphere and consider the trivial  -bundle
-bundle 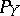 on
on 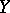 . Then the flat connections on
. Then the flat connections on 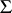 which extend to
which extend to 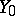 define a Lagrangian subspace
define a Lagrangian subspace  , and analogously
, and analogously 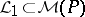 . Taking care of the singularity one may define
. Taking care of the singularity one may define 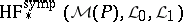 . The Atiyah–Floer conjecture reads
. The Atiyah–Floer conjecture reads
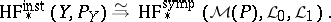 | (a1) |
This was originally conjectured by Atiyah in [a1]. An overview of the problem appears in [a8]. The problem is still open (as of 2000).
The symplectic Floer homology for a symplectic mapping was introduced by Floer in [a12]. Let 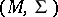 be a symplectic manifold which is monotone and simply connected. Let
be a symplectic manifold which is monotone and simply connected. Let 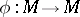 be a symplectomorphism. Then the symplectic Floer homology
be a symplectomorphism. Then the symplectic Floer homology  can be defined as the Morse-type theory where the critical points are the fixed points of
can be defined as the Morse-type theory where the critical points are the fixed points of 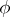 and the connecting orbits are
and the connecting orbits are 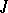 -holomorphic strips
-holomorphic strips 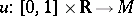 with
with 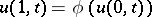 which converge to fixed points
which converge to fixed points 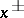 of
of 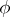 as
as 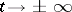 . For
. For 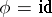 , Floer proved [a12] that
, Floer proved [a12] that  . Moreover, there is a natural ring structure for the symplectic Floer homology [a8], and in [a7] it is proved that there is an isomorphism of rings
. Moreover, there is a natural ring structure for the symplectic Floer homology [a8], and in [a7] it is proved that there is an isomorphism of rings 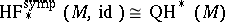 , where
, where 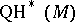 is the quantum cohomology of
is the quantum cohomology of 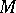 .
.
Let 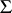 be a closed oriented surface of genus
be a closed oriented surface of genus 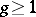 and let
and let  be the non-trivial
be the non-trivial 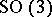 -bundle. The moduli space of flat connections
-bundle. The moduli space of flat connections 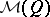 is a smooth symplectic manifold. Consider the mapping cylinder
is a smooth symplectic manifold. Consider the mapping cylinder 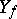 of a diffeomorphism
of a diffeomorphism 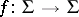 . This
. This 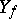 fibres over the circle
fibres over the circle 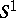 with fibre
with fibre 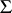 . Lift
. Lift  to a bundle mapping
to a bundle mapping 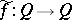 . This gives an
. This gives an 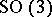 -bundle
-bundle 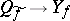 . On the other hand,
. On the other hand, 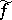 induces a mapping
induces a mapping  . The Atiyah–Floer conjecture for mapping cylinders was proposed by Floer [a4] and reads:
. The Atiyah–Floer conjecture for mapping cylinders was proposed by Floer [a4] and reads:
 | (a2) |
In [a3], Dostoglou and Salamon prove the existence of an isomorphism between these two Floer homologies by constructing an isomorphism at the chain level and identifying the boundary operators. The idea is named adiabatic limit and consists of stretching 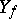 in the direction orthogonal to
in the direction orthogonal to 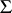 .
.
A very important case is that of 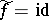 . Then
. Then 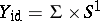 and
and  is the
is the 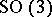 -bundle with
-bundle with 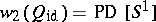 . Therefore,
. Therefore,
 | (a3) |
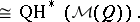 |
Both Floer homologies have natural product structures, introduced by S.K. Donaldson (see [a8]). A stronger version of the Atiyah–Floer conjecture establishes that (a3) is an isomorphism of rings.
The existence of such an isomorphism has been proved by V. Muñoz in [a5], [a6] by giving an explicit presentation of both rings in terms of the natural generators of the cohomology of 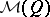 and using the relationship of instanton Floer homology of
and using the relationship of instanton Floer homology of  -manifolds with Donaldson invariants of
-manifolds with Donaldson invariants of 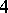 -manifolds [a2]. Also, in [a9] Salamon proves that the adiabatic limit isomorphism is indeed a ring isomorphism.
-manifolds [a2]. Also, in [a9] Salamon proves that the adiabatic limit isomorphism is indeed a ring isomorphism.
References
| [a1] | M.F. Atiyah, "New invariants of three and four dimensional manifolds" Proc. Symp. Pure Math. , 48 (1988) |
| [a2] | S.K. Donaldson, "On the work of Andreas Floer" Jahresber. Deutsch. Math. Verein. , 95 (1993) pp. 103–120 |
| [a3] | S. Dostoglou, D. Salamon, "Self-dual instantons and holomorphic curves" Ann. of Math. , 139 (1994) pp. 581–640 |
| [a4] | S. Dostoglou, D. Salamon, "Instanton homology and symplectic fixed points" D. Salamon (ed.) , Symplectic Geometry: Proc. Conf. , London Math. Soc. Lecture Notes , 192 , Cambridge Univ. Press (1993) pp. 57–94 |
| [a5] | V. Muñoz, "Ring structure of the Floer cohomology of  " Topology , 38 (1999) pp. 517–528 " Topology , 38 (1999) pp. 517–528 |
| [a6] | V. Muñoz, "Quantum cohomology of the moduli space of stable bundles over a Riemann surface" Duke Math. J. , 98 (1999) pp. 525–540 |
| [a7] | S. Piunikhin, D. Salamon, M. Schwarz, "Symplectic Floer–Donaldson theory and quantum cohomology" C.B. Thomas (ed.) , Contact and Symplectic Geometry , Publ. Newton Inst. , 8 , Cambridge Univ. Press (1996) pp. 171–200 |
| [a8] | D. Salamon, "Lagrangian intersections,  -manifolds with boundary and the Atiyah–Floer conjecture" , Proc. Internat. Congress Math. , 1 , Birkhäuser (1994) pp. 526–536 -manifolds with boundary and the Atiyah–Floer conjecture" , Proc. Internat. Congress Math. , 1 , Birkhäuser (1994) pp. 526–536 |
| [a9] | D. Salamon, "Quantum products for mapping tori and the Atiyah–Floer conjecture" Preprint ETH-Zürich (1999) |
| [a10] | A. Floer, "An instanton invariant for  -manifolds" Comm. Math. Phys. , 118 (1988) pp. 215–240 -manifolds" Comm. Math. Phys. , 118 (1988) pp. 215–240 |
| [a11] | A. Floer, "Symplectic fixed points and holomorphic spheres" Comm. Math. Phys. , 120 (1989) pp. 575–611 |
| [a12] | A. Floer, "Morse theory for the symplectic action" J. Diff. Geom. , 28 (1988) pp. 513–547 |
Atiyah-Floer conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Atiyah-Floer_conjecture&oldid=49962