Riesz operator

The Riesz operators on a Banach space are, roughly speaking, those bounded linear operators that have a Riesz spectral theory, i.e. that have a spectral theory like that of compact operators, [a8] (see also Spectral theory of compact operators).

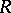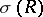The precise definition is as follows ([a2], [a5]). Let be a bounded operator on a Banach space , and let be the spectrum of 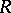. A point 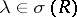is isolated if 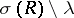is closed in 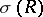, i.e. if there is an open subset 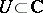such that 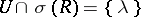. A point 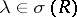is a Riesz point if it is isolated and 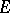is the direct sum of a closed subspace 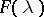and a finite-dimensional subspace , both invariant under 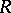and such that 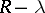is nilpotent on 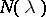and a homeomorphism on .

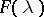A bounded operator 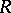is a Riesz operator if all points 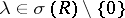are Riesz points. Every compact operator is a Riesz operator (the Riesz theory of compact operators).

A bounded operator on is called quasi-nilpotent if 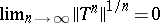(which is equivalent to ).

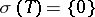A bounded operator 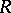is a Riesz operator if and only if, [a3]:

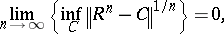where 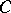runs over all compact operators (see Compact operator).

It is a long-standing question (still open as of 2000) whether every Riesz operator splits as the sum of a quasi-nilpotent operator and a compact operator. Such a decomposition is called a West decomposition, after T.T. West, who proved this for the case that 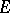is a Hilbert space, [a6]. Further results can be found in [a1], [a7].

There is another, quite different, notion in which the phrase "Riesz operator" occurs, viz. the parametrized family of multiplier operators

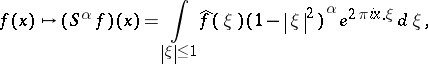called the Bochner–Riesz operator, [a4]. They are important in Bochner–Riesz summability (see also Riesz summation method).

References

[a1]	K.R. Davidson, D.A. Herrero, "Decomposition of Banach space operators" Indiana Univ. Math. J. , 35 (1986) pp. 333–343
[a2]	J. Dieudonné, "Foundations of modern analysis" , Acad. Press (1960) pp. 323
[a3]	A.F. Ruston, "Operators with a Fredholm theory" J. London Math. Soc. , 29 (1954) pp. 318–326
[a4]	E.M. Stein, "Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals" , Princeton Univ. Press (1993) pp. 389
[a5]	T.T. West, "Riesz operators in Banach spaces" Proc. London Math. Soc. , 16 (1966) pp. 131–140
[a6]	T.T. West, "The decomposition of Riesz operators" Proc. London Math. Soc. , 16 (1966) pp. 737–752
[a7]	H. Zhong, "On B-convex spaces and West decomposition of Riesz operators on them" Acta Math. Sinica , 37 (1994) pp. 563–569
[a8]	H.R. Dowson, "Spectral theory of linear operators" , Acad. Press (1978) pp. 67ff.