Daubechies wavelets
A wavelet is a function  that yields a basis in
that yields a basis in  by means of translations and dyadic dilations of itself, i.e.,
by means of translations and dyadic dilations of itself, i.e.,
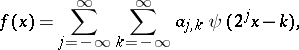 |
for all  (cf. also Wavelet analysis). Such a decomposition is called the discrete wavelet transform.
(cf. also Wavelet analysis). Such a decomposition is called the discrete wavelet transform.
In 1988, the Belgian mathematician I. Daubechies constructed [a2] a class of wavelet functions  ,
,  , that satisfy some special properties. First of all, the collection
, that satisfy some special properties. First of all, the collection  ,
,  , is an orthonormal system for fixed
, is an orthonormal system for fixed  . Furthermore, each wavelet
. Furthermore, each wavelet 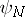 is compactly supported (cf. also Function of compact support). Moreover,
is compactly supported (cf. also Function of compact support). Moreover,  . The index number
. The index number  is also related to the number of vanishing moments, i.e.,
is also related to the number of vanishing moments, i.e.,
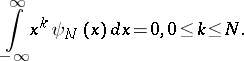 |
A last important property of the Daubechies wavelets is that their regularity increases linearly with their support width. In fact,
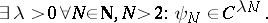 |
For large 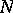 one has
one has 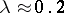 .
.
The Daubechies wavelets are neither symmetric nor anti-symmetric around any axis, except for 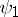 , which is in fact the Haar wavelet [a3]. Satisfying symmetry conditions cannot go together with all other properties of the Daubechies wavelets.
, which is in fact the Haar wavelet [a3]. Satisfying symmetry conditions cannot go together with all other properties of the Daubechies wavelets.
The Daubechies wavelets can also be used for the continuous wavelet transform, i.e.
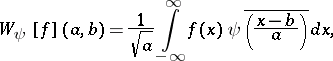 |
for 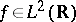 ,
,  and
and  . The parameters
. The parameters  and
and 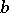 denote scale and translation/position of the transform. A stable reconstruction formula exists for the continuous wavelet transform if and only if the following admissibility condition holds:
denote scale and translation/position of the transform. A stable reconstruction formula exists for the continuous wavelet transform if and only if the following admissibility condition holds:
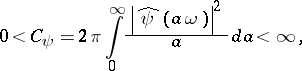 |
where  denotes the Fourier transform of
denotes the Fourier transform of 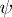 . The reconstruction formula reads:
. The reconstruction formula reads:
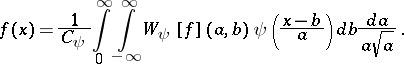 |
This result holds weakly in  . For
. For  and
and 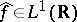 , this results also holds pointwise.
, this results also holds pointwise.
All Daubechies wavelets satisfy the admissibility condition and thus guarantee a stable reconstruction.
References
| [a1] | I. Daubechies, "Ten lectures on wavelets" , SIAM (1992) |
| [a2] | I. Daubechies, "Orthonormal bases of compactly supported wavelets" Commun. Pure Appl. Math. , 41 (1988) pp. 909–996 |
| [a3] | A. Haar, "Zur theorie der orthogonalen Funktionensysteme" Math. Ann. , 69 (1910) pp. 331–371 |
Daubechies wavelets. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Daubechies_wavelets&oldid=49909