Reflection

A mapping of an -dimensional simply-connected space of constant curvature (i.e. of a Euclidean or affine space , a sphere or a hyperbolic (Lobachevskii) space ) the set of fixed points of which is an -dimensional hyperplane. The set is called the mirror of the mapping ; in other words, is a reflection in . Every reflection is uniquely defined by its mirror. The order (period) of a reflection in the group of all motions of 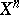is equal to 2, i.e. .

The Euclidean or affine space 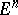can be identified with the vector space 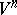of its parallel translations. The mapping 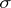is then a linear orthogonal transformation of 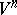with matrix

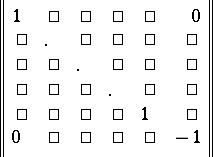in a certain orthonormal basis, and conversely, every orthogonal transformation of with this matrix in a certain orthonormal basis is a reflection in 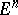. More generally, a linear transformation 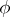of an arbitrary vector space 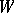over a field 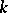, of characteristic other than 2, is called a linear reflection if 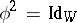and if the rank of the transformation 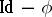is equal to 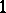. 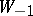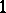In this case, the subspace of fixed vectors relative to has codimension in , the subspace of eigenvectors with eigenvalue has dimension and . 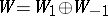If 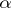is a linear form on such that 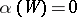when , and if 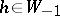is an element such that 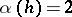, then is defined by the formula

The description of a reflection in an arbitrary simply-connected space 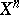of constant curvature can be reduced to the description of linear reflections in the following way. Every such space can be imbedded as a hypersurface in a real 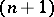-dimensional vector space in such a way that the motions of can be extended to linear transformations of 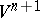. Moreover, in a suitable coordinate system in 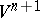the equations of the hypersurface can be written in the following way:

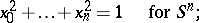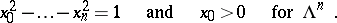Every hypersurface in 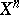, given this imbedding, is the intersection with 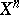of a certain -dimensional subspace in 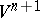, and every reflection in 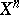is induced by a linear reflection in .

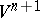If, in the definition of a linear reflection, the requirement that is dropped, then the more general concept of a pseudo-reflection is obtained. If 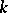is the field of complex numbers and 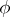is a pseudo-reflection of finite order (not necessarily equal to 2), then 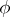is called a unitary reflection. Every biholomorphic automorphism of finite order of a bounded symmetric domain in a complex space the set of fixed points of which has a complex codimension 1 is also called a unitary reflection.

See also Reflection group.

References

[1]	N. Bourbaki, "Groupes et algèbres de Lie" , Eléments de mathématiques , Hermann (1968) pp. Chapts. 4–6
[2]	E.B. Vinberg, "Discrete linear groups generated by reflections" Math. USSR Izv. , 35 : 5 (1971) pp. 1083–1119 Izv. Akad. Nauk SSSR Ser. Mat. , 35 : 5 (1971) pp. 1072–1112
[3]	E. Gottschling, "Reflections in bounded symmetric domains" Comm. Pure Appl. Math. , 22 (1969) pp. 693–714
[4]	B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian)

Comments

The spelling reflexion also occurs in the literature.

A basic fact is that the reflections generate the orthogonal group; see [a2], Sects. 8.12.12, 13.7.12.

References

[a1]	B.A. [B.A. Rozenfel'd] Rosenfel'd, "A history of non-euclidean geometry" , Springer (1988) (Translated from Russian)
[a2]	M. Berger, "Geometry" , 1–2 , Springer (1987) (Translated from French)
[a3]	H.S.M. Coxeter, "Introduction to geometry" , Wiley (1963)
[a4]	M. Greenberg, "Euclidean and non-euclidean geometry" , Freeman (1980)
[a5]	B. Artmann, "Lineare Algebra" , Birkhäuser (1986)
[a6]	P.R. Halmos, "Finite-dimensional vector spaces" , v. Nostrand (1958)