Yamabe problem
Let 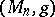 be a
be a 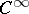 compact Riemannian manifold of dimension
compact Riemannian manifold of dimension 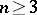 ; let
; let 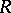 be its scalar curvature. The Yamabe problem is: Does there exist a metric
be its scalar curvature. The Yamabe problem is: Does there exist a metric 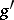 , conformal to
, conformal to  (cf. also Conformal-differential geometry), such that the scalar curvature
(cf. also Conformal-differential geometry), such that the scalar curvature 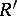 of
of 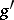 is constant?
is constant?
In 1960, H. Yamabe wanted to solve the Poincaré conjecture. As a first step he tried to make constant the scalar curvature by a conformal change of metrics. He thought he had succeeded. Unfortunately, in his beautiful paper [a15] there is a mistake in an inequality: 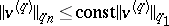 must be replaced by
must be replaced by  , and this does not yield the result in the general case. Now, thirty years afterwards, the problem is entirely solved.
, and this does not yield the result in the general case. Now, thirty years afterwards, the problem is entirely solved.
Yamabe was a pioneer of solving geometrical problems by analysis. If one writes the conformal deformation in the form 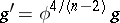 (where
(where 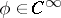 ,
,  ), then the scalar curvature
), then the scalar curvature 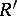 is given by
is given by
 |
with  . So, the Yamabe problem is equivalent to solving the equation
. So, the Yamabe problem is equivalent to solving the equation
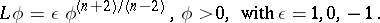 | (a1) |
Here, 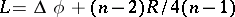 is the conformal Laplacian (cf. also Laplace operator). To solve this problem, Yamabe introduced the so-called Yamabe functional,
is the conformal Laplacian (cf. also Laplace operator). To solve this problem, Yamabe introduced the so-called Yamabe functional,  , with
, with  .
.
The Euler equation of 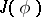 is (a1). Thus, the variational method seems applicable. Let
is (a1). Thus, the variational method seems applicable. Let 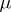 be the infimum of
be the infimum of 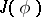 for
for 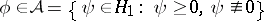 . One can prove that
. One can prove that 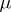 is a conformal invariant, and that
is a conformal invariant, and that 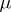 is the infimum of
is the infimum of  over all
over all 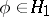 ,
, 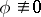 . But
. But 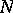 is the critical exponent in the Sobolev imbedding theorem (cf. Imbedding theorems), and
is the critical exponent in the Sobolev imbedding theorem (cf. Imbedding theorems), and 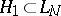 is not compact. Hence one cannot prove that
is not compact. Hence one cannot prove that 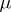 is attained. To overcome this difficulty, Yamabe considered the functionals
is attained. To overcome this difficulty, Yamabe considered the functionals  ,
, 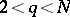 , and solved the family of approximated equations
, and solved the family of approximated equations
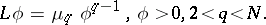 | (a2) |
The following theorem holds, [a15]: There exists a 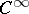 strictly positive function
strictly positive function  , with
, with 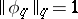 and satisfying equation (a2), where
and satisfying equation (a2), where 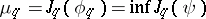 for all
for all  ,
,  .
.
According to the sign of 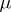 , there are three mutually exclusive cases: positive, negative and zero;
, there are three mutually exclusive cases: positive, negative and zero; 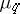 has the sign of
has the sign of  . Then Yamabe claimed that the set
. Then Yamabe claimed that the set 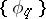 is uniformly bounded. This is not true on the sphere, and this cannot be overcome in the positive case. But in the negative case the wrong term plays no role, and one can remove it (it has negative sign). Yamabe's proof works also in the zero case: if
is uniformly bounded. This is not true on the sphere, and this cannot be overcome in the positive case. But in the negative case the wrong term plays no role, and one can remove it (it has negative sign). Yamabe's proof works also in the zero case: if 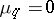 ,
, 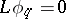 and
and  satisfies (a1) with
satisfies (a1) with  . In the positive case, if one considers the metric
. In the positive case, if one considers the metric  , then
, then 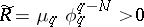 . So Yamabe was able to prove that there exists a conformal metric whose scalar curvature is either a non-positive constant or is everywhere positive.
. So Yamabe was able to prove that there exists a conformal metric whose scalar curvature is either a non-positive constant or is everywhere positive.
The positive case remained open. When 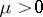 , one can exhibit subsequences
, one can exhibit subsequences  with
with  and
and  such that
such that 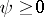 satisfies
satisfies 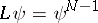 weakly in
weakly in 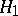 . However, there are two difficulties: the regularity and the triviality of
. However, there are two difficulties: the regularity and the triviality of 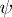 (according to the maximum principle, either
(according to the maximum principle, either  or
or 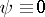 ). The regularity was resolved by N. Trudinger [a14]. He proved that a weak solution of (a1) is smooth. To prove that
). The regularity was resolved by N. Trudinger [a14]. He proved that a weak solution of (a1) is smooth. To prove that  is non-trivial, the best constants in the Sobolev imbedding theorem must be found. In [a1] (see also [a3]), T. Aubin considered three Banach spaces
is non-trivial, the best constants in the Sobolev imbedding theorem must be found. In [a1] (see also [a3]), T. Aubin considered three Banach spaces 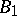 ,
, 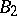 and
and 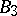 such that
such that  is continuous but not compact and
is continuous but not compact and 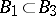 is compact. There are pairs of real numbers
is compact. There are pairs of real numbers 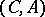 such that all
such that all  satisfy
satisfy 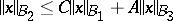 . It can be proved that
. It can be proved that 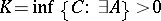 .
.
This situation occurs with 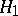 ,
,  and
and 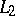 . Moreover, Aubin proved that the best constant
. Moreover, Aubin proved that the best constant  depends only on
depends only on  , and not upon the compact manifold. So, [a1], for any
, and not upon the compact manifold. So, [a1], for any  there exists a constant
there exists a constant 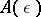 such that every
such that every 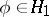 satisfies
satisfies 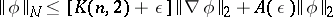 .
.
Recently it has been proved [a8] that the best constant is achieved (i.e., 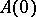 exists). Using the above result, Aubin was able to prove the key theorem [a2]:
exists). Using the above result, Aubin was able to prove the key theorem [a2]: 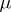 satisfies
satisfies  (for
(for 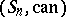 ,
,  ). If
). If 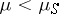 , then there exists a strictly positive
, then there exists a strictly positive  solution
solution 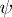 of
of  with
with 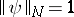 . For the metric
. For the metric 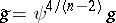 one has
one has 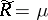 . Here,
. Here, 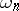 is the volume of
is the volume of 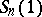 .
.
It remains to exhibit a test function 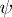 such that
such that 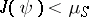 . All subsequent work has centred on the discovery of appropriate test functions.
. All subsequent work has centred on the discovery of appropriate test functions.
By considering the functional 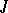 in a suitable conformal metric and using as test functions truncations of the functions
in a suitable conformal metric and using as test functions truncations of the functions 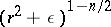 (here,
(here, 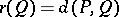 with
with 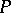 a point at which the Weyl tensor is not zero), Aubin was able to prove [a2] that if
a point at which the Weyl tensor is not zero), Aubin was able to prove [a2] that if 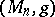 (
(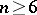 ) is a compact non-locally conformally flat Riemannian manifold, then
) is a compact non-locally conformally flat Riemannian manifold, then  . Hence there exists a conformal metric
. Hence there exists a conformal metric 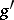 with
with 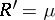 .
.
The remaining cases.
In 1984, G. Medrano [a6] proved that for a large class of locally conformally-flat manifolds 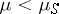 . At the same time, R. Schoen [a10] reduced the proof of
. At the same time, R. Schoen [a10] reduced the proof of 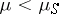 to the proof of the positive mass conjecture. If
to the proof of the positive mass conjecture. If 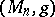 is locally conformally flat, there is a conformal metric
is locally conformally flat, there is a conformal metric 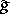 such that
such that 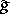 is flat in a neighbourhood
is flat in a neighbourhood  of
of 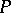 . In the positive case the conformal Laplacian is invertible; let
. In the positive case the conformal Laplacian is invertible; let  be its inverse. The expansion of
be its inverse. The expansion of 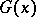 in
in 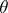 is
is
 | (a3) |
where 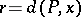 and
and 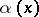 is a harmonic function.
is a harmonic function.
When 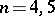 , in a conformal metric
, in a conformal metric 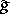 ,
,  has an expansion like (a3); when
has an expansion like (a3); when 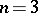 ,
, 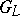 has the form (a3);
has the form (a3);  is
is 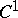 or Lipschitzian according to the dimension. In [a10] it is proved that if
or Lipschitzian according to the dimension. In [a10] it is proved that if 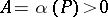 , then
, then 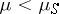 ; it uses test functions equal to
; it uses test functions equal to  in a neighbourhood of
in a neighbourhood of 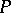 and equal to
and equal to 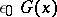 when
when  is large. It remained to proved that
is large. It remained to proved that 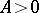 if
if 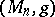 is not conformal to
is not conformal to  . This was done for
. This was done for 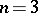 in [a12]. For locally conformally-flat manifolds the result is in [a13], and for dimensions smaller than
in [a12]. For locally conformally-flat manifolds the result is in [a13], and for dimensions smaller than 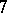 in [a11]. For a unification of the work of Aubin and Schoen, see [a9].
in [a11]. For a unification of the work of Aubin and Schoen, see [a9].
There are also direct proofs, not considering the functions  ; one proceeds by successive approximation, the other by the blow-up method. These proofs use the value of the best constant
; one proceeds by successive approximation, the other by the blow-up method. These proofs use the value of the best constant  . In [a4], A. Bahri presents an algebraic-topological proof for locally conformally-flat manifolds, not using the positive mass conjecture. Here it can not be shown that
. In [a4], A. Bahri presents an algebraic-topological proof for locally conformally-flat manifolds, not using the positive mass conjecture. Here it can not be shown that 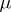 is achieved.
is achieved.
In [a7], both the Yamabe problem and the Lichnerowicz problem are solved.
Let 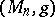 be a compact Riemannian manifold of dimension
be a compact Riemannian manifold of dimension  which is not conformal to
which is not conformal to  . There exists a conformal metric
. There exists a conformal metric 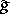 with
with 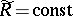 for which any conformal transformation is an isometric mapping
for which any conformal transformation is an isometric mapping 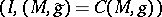 .
.
A generalization of the Yamabe problem is the prescribed scalar curvature problem in a given conformal class. This problem on  is known as the Nirenberg problem. Although research is intensive, these problems have not yet (1996) been entirely solved. On compact manifolds with boundary, P. Cherrier presented an original problem in [a5]: To find a conformal metric with prescribed scalar curvature and prescribed mean curvature of
is known as the Nirenberg problem. Although research is intensive, these problems have not yet (1996) been entirely solved. On compact manifolds with boundary, P. Cherrier presented an original problem in [a5]: To find a conformal metric with prescribed scalar curvature and prescribed mean curvature of 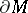 .
.
References
| [a1] | T. Aubin, "Espaces de Sobolev sur les variétés Riemanniennes" Bull. Sci. Math. , 100 (1976) pp. 149–173 |
| [a2] | T. Aubin, "Équations différentielles non-linéaires et problème de Yamabe concernant la courbure scalaire" J. Math. Pures Appl. , 55 (1976) pp. 269–296 |
| [a3] | T. Aubin, "Nonlinear analysis on manifolds. Monge–Ampère equations" , Springer (1982) |
| [a4] | A. Bahri, "Proof of the Yamabe conjecture, without the positive mass theorem, for locally conformally flat manifolds" T. Mabuchi (ed.) S. Mukai (ed.) , Einstein Metrics and Yang–Mills Connections , M. Dekker (1993) |
| [a5] | P. Cherrier, "Problèmes de Neumann non-linéaires sur les variétés Riemanniennes" J. Funct. Anal. , 57 (1984) pp. 154–206 |
| [a6] | O.G. Medrano, "On the Yamabe problem concerning the compact locally conformally flat manifolds" J. Funct. Anal. , 66 (1986) pp. 42–53 |
| [a7] | E. Hebey, M Vaugon, "Le problème de Yamabe équivariant" Bull. Sci. Math. , 117 (1993) pp. 241–286 |
| [a8] | E. Hebey, M. Vaugon, "The best constant problem in the Sobolev embedding theorem for complete Riemannian manifolds" Duke Math. J. , 79 (1995) pp. 235–279 |
| [a9] | J.M. Lee, T.H. Parker, "The Yamabe problem" Bull. Amer. Math. Soc. , 17 (1987) pp. 37–91 |
| [a10] | R. Schoen, "Conformal deformation of a Riemannian metric to constant scalar curvature" J. Diff. Geom. , 20 (1984) pp. 479–495 |
| [a11] | R. Schoen, "Variational theory for the total scalar curvature functional for Riemannian metrics and related topics" , Topics in Calculus of Variations , Lecture Notes in Mathematics , 1365 , Springer (1989) |
| [a12] | R. Schoen, S.T. Yau, "On the proof of the positive mass conjecture in general relativity" Comm. Math. Phys. , 65 (1979) pp. 45–76 |
| [a13] | R. Schoen, S.T. Yau, "Conformally flat manifolds, Kleinian groups and scalar curvature" Invent. Math. , 92 (1988) pp. 47–71 |
| [a14] | N. Trudinger, "Remarks concerning the conformal deformation of Riemannian structures on compact manifolds" Ann. Scuola Norm. Sup. Pisa , 22 (1968) pp. 265–274 |
| [a15] | H. Yamabe, "On a deformation of Riemannian strctures on compact manifolds" Osaka Math. J. , 12 (1960) pp. 21–37 |
Yamabe problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Yamabe_problem&oldid=49239