Volterra series

integro-power series

A series containing the powers of the unknown function under the integral sign. Let be a continuous function in all variables in a cube and let be an arbitrary continuous function on . The expression

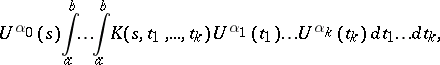where are non-negative integers and , is called a Volterra term of degree in . Two Volterra terms of degree 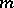belong to the same type if they differ only in their kernels 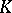. The finite sum of Volterra terms (of all types) of degree is called a Volterra form of degree in the function . It is denoted by

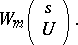Let

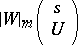denote the Volterra form in which the kernel 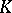is replaced by 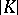, and let

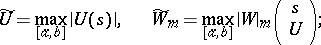then

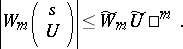The expression

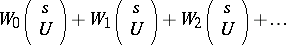is called a Volterra series. If the series of numbers converges, then the Volterra series is called regularly convergent. In this case the Volterra series converges absolutely and uniformly, and its sum is continuous on .

Analogously one introduces Volterra series in several functional arguments, and Volterra series in which is replaced by some closed bounded set in a finite-dimensional Euclidean space. Volterra series are a particular case of the more general concept of an abstract power series.

References

[1]	A.M. Lyapunov, "On equilibrium figures deviating slightly from ellipsoids of rotation of homogeneous fluid masses" , Collected Works , 4 , Moscow (1959) (In Russian)
[2]	E. Schmidt, "Zur Theorie der linearen und nichtlinearen Integralgleichungen III" Math. Ann. , 65 (1908) pp. 370–399
[3]	M.M. Vainberg, V.A. Trenogin, "Theory of branching of solutions of non-linear equations" , Noordhoff (1974) (Translated from Russian)

Comments

A non-linear input-output dynamical system with input and output gives rise to a Volterra series of the form

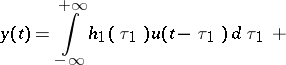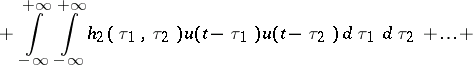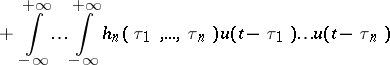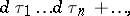in which if for some . Such series were first introduced by V. Volterra, [a1], and first applied to questions of system theory by N. Wiener, leading to Wiener integrals, [a2]. Cf. [a3] for an extensive discussion of Volterra series in system theory.

References

[a1]	V. Volterra, "Theory of functionals and of integral and integro-differential equations" , Dover, reprint (1959) (Translated from French)
[a2]	N. Wiener, "Nonlinear problems in random theory" , M.I.T. (1958)
[a3]	M. Schetzen, "The Volterra and Wiener theories of nonlinear systems" , Wiley (1980)