VMO-space

space of functions of vanishing mean oscillation

The class of functions of vanishing mean oscillation on , denoted by , is the subclass of consisting of the functions with the property that

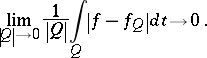Here, denotes the volume of the ball and denotes the mean of over 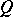(see -space). As with 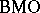, can be defined for spaces of homogeneous type.

Some properties of are as follows (see also [a1], [a2], [a3]). Bounded, uniformly continuous functions are in (cf. Uniform continuity), and 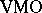can be obtained as the 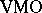-closure of the continuous functions that vanish at infinity. The Hilbert transform of a bounded, uniformly continuous function on is in . 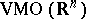is the dual of the Hardy space 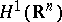(cf. also Hardy spaces).

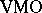appears in the theory of Douglas algebras: Let 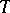be the boundary of the unit disc in . Let denote the subspace of 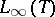consisting of the boundary values of bounded holomorphic functions (cf. Analytic function) on the unit disc and let 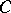denote the set of continuous functions on 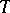. Put 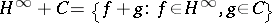. 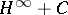is a closed subalgebra of 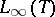and the simplest example of a Douglas algebra. Its largest self-adjoint subalgebra, 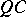, equals 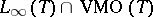, [a2], [a3].

See also -space.

References

[a1]	R.R. Coifman, G. Weiss, "Extensions of Hardy-spaces and their use in analysis" Bull. Amer. Math. Soc. , 83 (1977) pp. 569–645
[a2]	J. Garnett, "Bounded analytic functions" , Acad. Press (1981)
[a3]	D. Sarason, "Functions of vanishing mean oscillation" Trans. Amer. Math. Soc. , 207 (1975) pp. 391–405