Symplectic connection

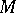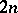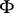An affine connection on a smooth manifold of dimension with a non-degenerate -form that is covariantly constant with respect to it. If the affine connection on 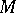is given by the local connection forms

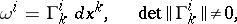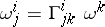and

then the condition that 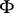be covariantly constant can be expressed in the form

The -form 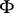defines a symplectic (or almost-Hamiltonian) structure on 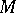that converts every tangent space 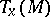into a symplectic space with the skew-symmetric scalar product 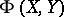. A symplectic connection can also be defined as an affine connection on 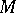which preserves this product under parallel transfer of vectors. In every 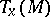one can choose a frame such that

The set of all such frames forms a principal fibre bundle over , whose structure group is the symplectic group. A symplectic connection is just a connection in this principal fibre bundle. There are manifolds 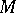of even dimension on which there is no non-degenerate globally defined -form 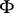and, consequently, no symplectic connection. However, if 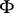exists, a symplectic connection with respect to which 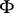is covariantly constant is not uniquely determined.

References

[1]	S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964)

References

[a1]	R. Abraham, J.E. Marsden, "Foundations of mechanics" , Benjamin/Cummings (1978)