Summability multipliers

Numerical factors (for the terms of a series) that transform a series

(1)

which is summable by a summation method (cf. Summation methods) into a series

(2)

which is summable by a method . In this case, the summability multipliers are called summability multipliers of type . For example, the numbers are summability multipliers of type 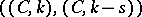(see Cesàro summation methods) when 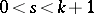(see [1]).

The fundamental problem in the theory of summability multipliers is to find conditions under which numbers 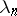will be summability multipliers of one type or another. This question is formulated more exactly in the following way: If 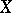and 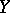are two classes of series, then what conditions have to be imposed on the numbers so that for every series (1) from 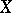, the series (2) belongs to 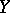? The appearance of the theory of summability multipliers goes back to the Dedekind–Hadamard theorem: The series (2) converges for any convergent series (1) if and only if

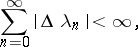where 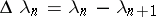. There is a generalization of this theorem with summability by the Cesàro method.

References

[1]	G.H. Hardy, "Divergent series" , Clarendon Press (1949)
[2]	G.F. Kangro, "On summability factors" Uchen. Zapiski Tartusk. Univ. , 37 (1955) pp. 191–232 (In Russian)
[3]	G.F. Kangro, "Theory of summability of sequences and series" J. Soviet Math. , 5 : 1 (1970) pp. 1–45 Itogi Nauk. i Tekhn. Mat. Anal. , 12 (1974) pp. 5–70
[4]	S.A. Baron, "Introduction to the theory of summability of series" , Tartu (1966) (In Russian)
[5]	C.N. Moore, "Summable series and convergence factors" , Dover, reprint (1966)