Sturm theorem

If

(*)

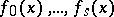is a Sturm series on the interval , , and is the number of variations of sign in the series (*) at a point (vanishing terms are not taken into consideration), then the number of distinct roots of the function on the interval is equal to the difference .

A Sturm series (or Sturm sequence) is a sequence of real-valued continuous functions (*) on having a finite number of roots on this interval, and such that

1) ;

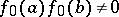2) 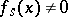on ;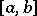

3) from 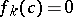for some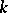 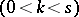and given in 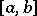it follows that ;

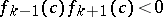4) from 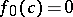for a given it follows that for sufficiently small ,

This theorem was proved by J.Ch. Sturm [1], who also proposed the following method of constructing a Sturm series for a polynomial 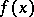with real coefficients and without multiple roots: 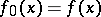, , 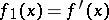and, if the polynomials 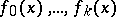are already constructed, then as 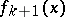one should take minus the remainder occurring in the process of dividing 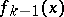by 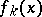. Here, will be a non-zero constant.

References

[1]	J.Ch. Sturm, Bull. de Férussac , 11 (1829)
[2]	A.G. Kurosh, "Higher algebra" , MIR (1972) (Translated from Russian)

Comments

The coefficients of the polynomials in the Sturm series must belong to a real-closed field. The algorithm to determine a Sturm series for a polynomial can be described as follows:

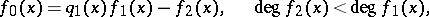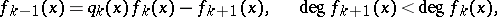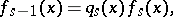so is a non-zero constant.

References

[a1]	N. Jacobson, "Basic algebra" , I , Freeman (1974)
[a2]	L.E.J. Dickson, "New first course in the theory of equations" , Wiley (1939)
[a3]	B.L. van der Waerden, "Algebra" , 1 , Springer (1967) (Translated from German)