Stone lattice
A pseudo-complemented distributive lattice  (see Lattice with complements) in which
(see Lattice with complements) in which 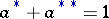 for all
for all 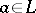 . A pseudo-complemented distributive lattice
. A pseudo-complemented distributive lattice 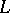 is a Stone lattice if and only if the join of any two of its minimal prime ideals is the whole of
is a Stone lattice if and only if the join of any two of its minimal prime ideals is the whole of 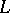 (the Grätzer–Schmidt theorem, [3]).
(the Grätzer–Schmidt theorem, [3]).
A Stone lattice, considered as a universal algebra with the basic operations  , is called a Stone algebra. Every Stone algebra is a subdirect product of two-element and three-element chains. In a pseudo-complemented lattice, an element
, is called a Stone algebra. Every Stone algebra is a subdirect product of two-element and three-element chains. In a pseudo-complemented lattice, an element  is said to be dense if
is said to be dense if 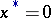 . The centre
. The centre 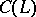 of a Stone lattice
of a Stone lattice 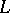 (cf. Centre of a partially ordered set) is a Boolean algebra, while the set
(cf. Centre of a partially ordered set) is a Boolean algebra, while the set 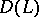 of all its dense elements is a distributive lattice with a unit. Moreover, there is a homomorphism
of all its dense elements is a distributive lattice with a unit. Moreover, there is a homomorphism  from
from 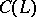 into the lattice
into the lattice 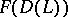 of filters of
of filters of 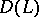 , defined by
, defined by
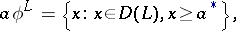 |
which preserves 0 and 1.
The triplet 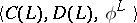 is said to be associated with the Stone algebra
is said to be associated with the Stone algebra 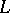 . Homomorphisms and isomorphisms of triplets are defined naturally. Any triplet
. Homomorphisms and isomorphisms of triplets are defined naturally. Any triplet 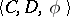 , where
, where 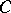 is a Boolean algebra,
is a Boolean algebra, 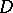 is a distributive lattice with a
is a distributive lattice with a 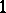 and
and 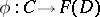 is a homomorphism preserving 0 and 1, is isomorphic to the triplet associated with some Stone algebra. Stone algebras are isomorphic if and only if their associated triplets are isomorphic (the Chen–Grätzer theorem, [2]).
is a homomorphism preserving 0 and 1, is isomorphic to the triplet associated with some Stone algebra. Stone algebras are isomorphic if and only if their associated triplets are isomorphic (the Chen–Grätzer theorem, [2]).
References
| [1] | G. Birkhoff, "Lattice theory" , Colloq. Publ. , 25 , Amer. Math. Soc. (1973) |
| [2] | C.C. Chen, G. Grätzer, "Stone lattices I-II" Canad. J. Math. , 21 : 4 (1969) pp. 884–903 |
| [3] | G. Grätzer, E.T. Schmidt, "On a problem of M.H. Stone" Acta Math. Acad. Sci. Hung. , 8 : 3–4 (1957) pp. 455–460 |
| [4] | T.S. Fofanova, "General theory of lattices" , Ordered sets and lattices , 3 , Saratov (1975) pp. 22–40 (In Russian) |
Comments
Stone lattices occur, in particular, as the open-set lattices of extremally-disconnected spaces (see Extremally-disconnected space), and are so named in honour of M.H. Stone's investigation of such spaces [a1]. If 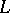 is the lattice of all open sets of a compact extremally-disconnected space
is the lattice of all open sets of a compact extremally-disconnected space 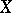 , then
, then 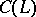 is a complete Boolean algebra, and
is a complete Boolean algebra, and 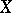 is its Stone space; thus, in this case
is its Stone space; thus, in this case 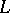 is entirely determined by
is entirely determined by  .
.
References
| [a1] | M.H. Stone, "Algebraic characterization of special Boolean rings" Fund. Math. , 29 (1937) pp. 223–303 |
| [a2] | G. Grätzer, "General lattice theory" , Birkhäuser (1978) (Original: Lattice theory. First concepts and distributive lattices. Freeman, 1978) |
Stone lattice. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Stone_lattice&oldid=48866