Spectral density

of a stationary stochastic process or of a homogeneous random field in -dimensional space

The Fourier transform of the covariance function of a stochastic process which is stationary in the wide sense (cf. Stationary stochastic process; Random field, homogeneous). Stationary stochastic processes and homogeneous random fields for which the Fourier transform of the covariance function exists are called processes with a spectral density.

Let

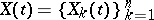be an -dimensional stationary stochastic process, and let

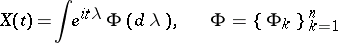be its spectral representation (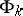 is the spectral measure corresponding to the 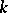-th component 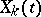of the multi-dimensional stochastic process 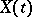). The range of integration is in the case of discrete time 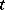, and 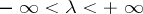in the case of continuous time . The process 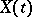has a spectral density

if all the elements

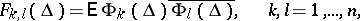of the spectral measure 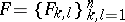are absolutely continuous and if

In particular, if the relation

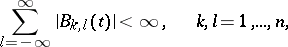holds for 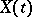, 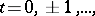where

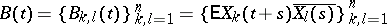is the covariance function of , then 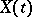has a spectral density and

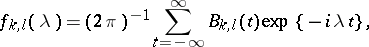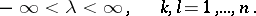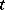The situation is similar in the case of processes in continuous time . The spectral density 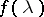is sometimes called the second-order spectral density, in contrast to higher spectral densities (see Spectral semi-invariant).

A homogeneous -dimensional random field 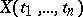has a spectral density 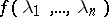if its spectral resolution 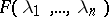possesses the property that its mixed derivative 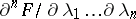exists almost-everywhere, and then

and

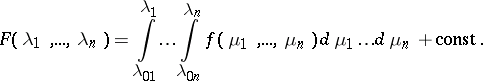References

[1]	Yu.V. [Yu.V. Prokhorov] Prohorov, Yu.A. Rozanov, "Probability theory, basic concepts. Limit theorems, random processes" , Springer (1969) (Translated from Russian)
[2]	Yu.A. Rozanov, "Stationary random processes" , Holden-Day (1967) (Translated from Russian)