Singular solution

of an ordinary differential equation

A solution at every point of which the uniqueness of the solution of the Cauchy problem for this equation is violated. For example, for an equation of the first order

(*)

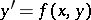with a continuous right-hand side which has a finite or infinite partial derivative everywhere with respect to 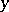, a singular solution can only lie in the set

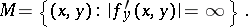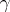A curve is a singular solution of (*) if is an integral curve of the equation (*) and if at least one more integral curve of (*) passes through every point of 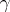. Let equation (*) have a general integral in a domain 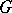; if this family of curves has an envelope, then this is a singular solution of equation (*). For a differential equation

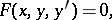a singular solution is found by examining the discriminant curve.

References

[1]	W.W. [V.V. Stepanov] Stepanow, "Lehrbuch der Differentialgleichungen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian)
[2]	G. Sansone, "Ordinary differential equations" , 2 , Zanichelli (1948) (In Italian)

Comments

Under "singular solution of a differential equation" is also understood a particular solution that is not obtainable by specifying the integration constant in a general solution. The two notions have much to do with one another but are not identical, cf. [a1].

References

[a1]	E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956) pp. §§3.6, 3.51, 4.7, A.5