Given an open subset  of
of  with smooth boundary
with smooth boundary  and
and  an
an  function, the Signorini problem consists in finding a function
function, the Signorini problem consists in finding a function  on
on  that is a solution to the following boundary value problem:
that is a solution to the following boundary value problem:
Here,  is a second-order linear and symmetric elliptic operator on
is a second-order linear and symmetric elliptic operator on  (in particular,
(in particular, 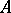 can be equal to
can be equal to  , the Laplace operator) and
, the Laplace operator) and  is the outward normal derivative to
is the outward normal derivative to  corresponding to
corresponding to  . This problem, introduced by A. Signorini [a5] and studied first by G. Fichera [a3], describes the mathematical model for the deformation of an elastic body whose boundary is in unilateral contact with another elastic body (the static case). In this case
. This problem, introduced by A. Signorini [a5] and studied first by G. Fichera [a3], describes the mathematical model for the deformation of an elastic body whose boundary is in unilateral contact with another elastic body (the static case). In this case 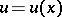 is the field of displacements and
is the field of displacements and  is the normal stress (see [a2]). In the Signorini problem, the boundary conditions can be equivalently expressed as:
is the normal stress (see [a2]). In the Signorini problem, the boundary conditions can be equivalently expressed as:
where  is an unknown part of
is an unknown part of  . Thus, the Signorini problem can be viewed as a problem with free boundary, and a weak formulation of the problem is given by the variational inequality [a4]:
. Thus, the Signorini problem can be viewed as a problem with free boundary, and a weak formulation of the problem is given by the variational inequality [a4]:
where  is the Dirichlet bilinear form associated to
is the Dirichlet bilinear form associated to  and
and  . Here,
. Here,  is the usual Sobolev space on
is the usual Sobolev space on  . In this way the existence and uniqueness of a weak solution to the Signorini problem follows from the general theory of elliptic variational inequalities (see [a1], [a4]).
. In this way the existence and uniqueness of a weak solution to the Signorini problem follows from the general theory of elliptic variational inequalities (see [a1], [a4]).
References
| [a1] | H. Brezis, "Inéquations variationelles" J. Math. Pures Appl. , 51 (1972) pp. 1–168 |
| [a2] | G. Duvaut, J.L. Lions, "Inequalities in mechanics and physics" , Springer (1976) |
| [a3] | G. Fichera, "Problemi elastostatici con vincoli unilaterali e il problema di Signorini con ambigue condizioni al contorno" Memoirs Acad. Naz. Lincei , 8 (1964) pp. 91–140 |
| [a4] | J.L. Lions, G. Stampacchia, "Variational inequalities" Comm. Pure Appl. Math. , XX (1967) pp. 493–519 |
| [a5] | A. Signorini, "Questioni di elastostatica linearizzata e semilinearizzata" Rend. Mat. Appl. , XVIII (1959) |
How to Cite This Entry:
Signorini problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Signorini_problem&oldid=48698
This article was adapted from an original article by V. Barbu (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article of
of  with smooth boundary
with smooth boundary  and
and  an
an  function, the Signorini problem consists in finding a function
function, the Signorini problem consists in finding a function  on
on  that is a solution to the following boundary value problem:
that is a solution to the following boundary value problem:
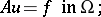
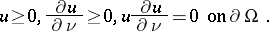
 is a second-order linear and symmetric elliptic operator on
is a second-order linear and symmetric elliptic operator on  (in particular,
(in particular, 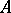 can be equal to
can be equal to  , the Laplace operator) and
, the Laplace operator) and  is the outward normal derivative to
is the outward normal derivative to  corresponding to
corresponding to  . This problem, introduced by A. Signorini [a5] and studied first by G. Fichera [a3], describes the mathematical model for the deformation of an elastic body whose boundary is in unilateral contact with another elastic body (the static case). In this case
. This problem, introduced by A. Signorini [a5] and studied first by G. Fichera [a3], describes the mathematical model for the deformation of an elastic body whose boundary is in unilateral contact with another elastic body (the static case). In this case 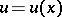 is the field of displacements and
is the field of displacements and  is the normal stress (see [a2]). In the Signorini problem, the boundary conditions can be equivalently expressed as:
is the normal stress (see [a2]). In the Signorini problem, the boundary conditions can be equivalently expressed as:
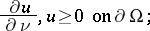

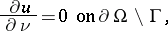
 is an unknown part of
is an unknown part of  . Thus, the Signorini problem can be viewed as a problem with free boundary, and a weak formulation of the problem is given by the variational inequality [a4]:
. Thus, the Signorini problem can be viewed as a problem with free boundary, and a weak formulation of the problem is given by the variational inequality [a4]:
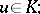

 is the Dirichlet bilinear form associated to
is the Dirichlet bilinear form associated to  and
and  . Here,
. Here,  is the usual Sobolev space on
is the usual Sobolev space on  . In this way the existence and uniqueness of a weak solution to the Signorini problem follows from the general theory of elliptic variational inequalities (see [a1], [a4]).
. In this way the existence and uniqueness of a weak solution to the Signorini problem follows from the general theory of elliptic variational inequalities (see [a1], [a4]).