Serial subgroup
From Encyclopedia of Mathematics
Let  be a subgroup of a group
be a subgroup of a group 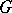 . A series of subgroups between
. A series of subgroups between 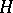 and
and 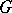 , or, more briefly, a series between
, or, more briefly, a series between 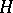 and
and 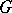 , is a set of subgroups of
, is a set of subgroups of 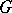 ,
,
 |
where  is a linearly ordered set, such that
is a linearly ordered set, such that
i) 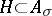 ,
, 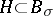 for all
for all  ;
;
ii) 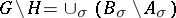 ;
;
iii) 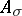 is a normal subgroup of
is a normal subgroup of 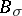 ;
;
iv) 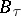 is a subgroup of
is a subgroup of 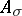 if
if  .
.
It follows that for all 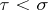 ,
,
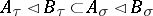 |
and
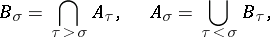 |
and for a finite series, indexed by 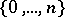 , hence
, hence
 |
A subgroup 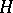 is called serial if there is a series of subgroups between
is called serial if there is a series of subgroups between  and
and  . If
. If 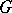 is finite, a subgroup
is finite, a subgroup 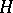 is serial if and only if it is a subnormal subgroup. A subgroup
is serial if and only if it is a subnormal subgroup. A subgroup 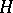 is called an ascendant subgroup in
is called an ascendant subgroup in  if there is an ascending series of subgroups between
if there is an ascending series of subgroups between 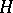 and
and 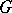 , that is, a series whose index set
, that is, a series whose index set  is well-ordered.
is well-ordered.
References
| [a1] | D.J.S. Robinson, "Finiteness condition and generalized soluble groups" , 1 , Springer (1972) pp. Chapt. 1 |
How to Cite This Entry:
Serial subgroup. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Serial_subgroup&oldid=48677
Serial subgroup. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Serial_subgroup&oldid=48677