Separability of sets

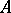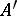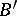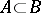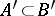A basic concept in descriptive set theory (introduced by N.N. Luzin [1]). It is an important instrument in the study of the descriptive nature of sets. Two sets and are said to be separable by sets possessing a property if there exist two sets and possessing property such that , and .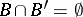

The first results on separability were obtained by Luzin and P.S. Novikov. Many variants of separability theorems appeared later, and the actual concept of separability of sets was generalized and given new forms. One such generalization is embodied by Novikov's theorem [2]: Let 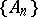be a sequence of 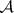-sets (cf. -set) in a complete separable metric space such that 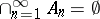. Then there is a sequence of Borel sets (cf. 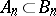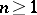Borel set) such that , , and 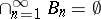. This theorem and some of its variants and generalizations are called theorems of multiple (or generalized) separability.

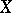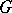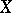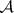The classical results relate to sets in complete separable metric spaces. 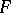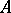In a Hausdorff space : 1) two disjoint analytic sets are separable by Borel sets generated by the system of open sets of this space [3] (if is a Urysohn space, then "open sets G" can be replaced by "closed sets F" ; in a Hausdorff space, generally speaking, this cannot be done [4]); 2) let be the system of -sets generated by a system ; if is an 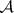-set generated by the system 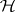and is an analytic set, , 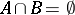then there is a Borel set 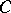generated by 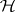such that 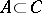, 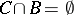(see [5]).

In contrast to these and other variants of the first separation principle, many formulations of the second separation principle do not depend on the topology of the space in which the sets are situated. One formulation is as follows [6]: Let a system 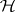of subsets of a given set be closed with respect to the operation of transfer to the complement and let it contain 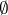; let 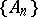be an arbitrary sequence of -sets (cf. 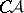-set) generated by 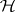; then there is a sequence 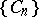of pairwise disjoint 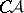-sets generated by 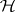such that 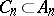, , 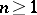and 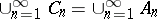(more accurately, this is one of the formulations of the reduction principle, see [7]).

References

[1]	N.N. [N.N. Luzin] Lusin, "Leçons sur les ensembles analytiques et leurs applications" , Gauthier-Villars (1930)
[2]	P.S. Novikov, "On the countable separability of analytic sets" Dokl. Akad. Nauk SSSR , 3–4 : 3 (1934) pp. 145–149 (In Russian) (French abstract)
[3]	Z. Frolik, "A survey of separable desciptive theory of sets and spaces" Czechoslovak. Math. J. , 20 (1970) pp. 406–467
[4]	A.J. Ostaszewski, "On Luzin's separation principles in Hausdorff spaces" Proc. London Math. Soc. , 27 : 4 (1973) pp. 649–666
[5]	C.A. Rogers, "Luzin's first separation axiom" J. London Math. Soc. , 3 : 1 (1971) pp. 103–108
[6]	C.A. Rogers, "Luzin's second separation theorem" J. London Math. Soc. , 6 : 3 (1973) pp. 491–503
[7]	K. Kuratowski, "Topology" , 1 , Acad. Press (1966) (Translated from French)

References

[a1]	T.J. Jech, "Set theory" , Acad. Press (1978) pp. 523ff (Translated from German)