Semi-symplectic space
A projective 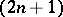 -space in which there is given a
-space in which there is given a 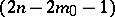 -plane
-plane 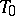 , in this a
, in this a 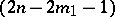 -plane
-plane 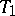 , etc., up to a
, etc., up to a 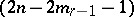 -plane
-plane 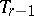 , where in the space a null-system is given, taking all the points of the space to planes passing through the plane
, where in the space a null-system is given, taking all the points of the space to planes passing through the plane 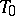 ; the plane
; the plane 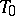 is given an absolute null-system taking all its points to
is given an absolute null-system taking all its points to 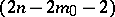 -planes lying in it and passing through the
-planes lying in it and passing through the 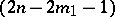 -plane
-plane 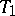 , etc., up to an absolute null-system of the
, etc., up to an absolute null-system of the 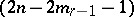 -plane
-plane 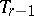 , taking all its points to
, taking all its points to 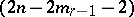 -planes lying in it,
-planes lying in it, 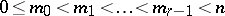 . This semi-symplectic space is denoted by
. This semi-symplectic space is denoted by 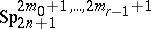 .
.
A semi-symplectic space is obtained by a method analogous to the transition from elliptic and hyperbolic spaces to semi-elliptic and semi-hyperbolic spaces, and is more general than a quasi-symplectic space.
The collineations of a semi-symplectic space that take the planes 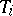 to themselves and that commute with the null-systems are called semi-symplectic transformations of the semi-symplectic space.
to themselves and that commute with the null-systems are called semi-symplectic transformations of the semi-symplectic space.
There exist invariants of semi-symplectic transformations analogous to the symplectic invariants of symplectic spaces. The semi-symplectic transformations form a Lie group.
References
| [1] | B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian) |
Comments
References
| [a1] | B.A. [B.A. Rozenfel'd] Rosenfel'd, "A history of non-euclidean geometry" , Springer (1988) (Translated from Russian) |
Semi-symplectic space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Semi-symplectic_space&oldid=48667