Semi-pseudo-Riemannian space

A manifold with a degenerate indefinite metric. The semi-pseudo-Riemannian manifold 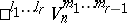is defined as an -dimensional manifold with coordinates 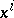in which there are given line elements

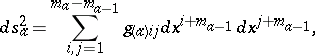where 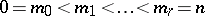; ; 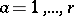and where the index of the quadratic form 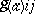is 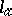. The line element 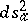is defined for those vectors for which all components with indices smaller than 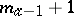or larger than 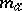vanish. If 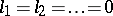, a semi-pseudo-Riemannian space is a semi-Riemannian space. The spaces 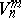and 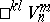are quasi-Riemannian spaces. The basic concepts of differential geometry (for example, curvature) are defined in semi-pseudo-Riemannian spaces similarly to Riemannian spaces (see [1]).

References

[1]	B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian)

References

[a1]	B.A. [B.A. Rozenfel'd] Rosenfel'd, "A history of non-euclidean geometry" , Springer (1988) (Translated from Russian)