Schwarz symmetric derivative
From Encyclopedia of Mathematics
of a function 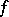 at a point
at a point 
The value
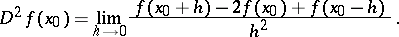 |
It is sometimes called the Riemann derivative or the second symmetric derivative. For the first time introduced by B. Riemann in 1854 (see [2]); it was studied by H.A. Schwarz [1]. More generally, the symmetric derivative of order  is also called a Schwarz symmetric derivative:
is also called a Schwarz symmetric derivative:
 |
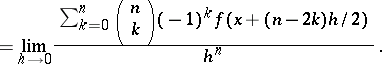 |
References
| [1] | H.A. Schwarz, "Beweis eines für die Theorie der trigonometrischen Reihen in Betracht kommenden Hülfssatzes" , Gesammelte Math. Abhandlungen , Chelsea, reprint (1972) pp. 341–343 |
| [2] | B. Riemann, "Ueber die Darstellbarkeit einer Function durch eine trigonometrische Reihe" H. Weber (ed.) , B. Riemann's Gesammelte Mathematische Werke , Dover, reprint (1953) pp. 227–271 ((Original: Göttinger Akad. Abh.  (1868))) (1868))) |
| [3] | I.P. Natanson, "Theory of functions of a real variable" , 1–2 , F. Ungar (1955–1961) (Translated from Russian) |
| [4] | N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian) |
| [5] | A. Zygmund, "Trigonometric series" , 1–2 , Cambridge Univ. Press (1988) |
Comments
The name general derivative is also used for this notion. A natural approach is to start with the central difference 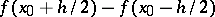 , and to define the first symmetric derivative as
, and to define the first symmetric derivative as
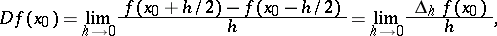 |
and then 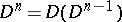 ,
, 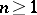 ,
, 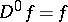 .
.
References
| [a1] | W. Rudin, "Real and complex analysis" , McGraw-Hill (1974) pp. 24 |
How to Cite This Entry:
Schwarz symmetric derivative. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schwarz_symmetric_derivative&oldid=48635
Schwarz symmetric derivative. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schwarz_symmetric_derivative&oldid=48635
This article was adapted from an original article by T.P. Lukashenko (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article