Saddle node

A type of arrangement of the trajectories in a neighbourhood of a singular point 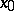of an autonomous system of second-order ordinary differential equations

(*)

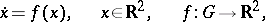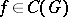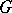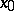, where is the domain of uniqueness. This type is characterized as follows. Suppose that a certain neighbourhood of is partitioned into (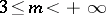) curvilinear sectors (cf. Sector in the theory of ordinary differential equations) by semi-trajectories (the separatrices of the saddle node) approaching 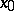. Suppose that 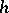of these sectors, 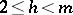, are saddle sectors and that the others are open nodal sectors, and suppose also that each semi-trajectory approaching 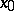, completed with 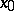, touches it in a definite direction. Then 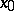is called a saddle node.

A saddle node is unstable in the sense of Lyapunov (cf. Lyapunov stability). Its Poincaré index is 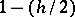(cf. Singular point). If 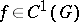and the matrix , then the singular point 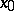can be a saddle node for (*) only when the eigenvalues 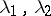of 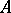satisfy one of the following conditions:

a) ;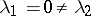

b) .

In any of these cases can also be a saddle or a node for (*), and in case b), also a point of another type. If it is a saddle node, then 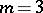, , 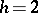and all the semi-trajectories of the system that approach 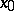touch at this point the directions defined by the eigenvectors of 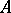(see Fig. aand Fig. b, where the heavy lines are the separatrices at the saddle node , and the arrows indicate the direction of motion along the trajectories of the system as 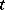increases; they can also be in the opposite direction).

Figure: s083040a

Figure: s083040b

References

[1]	N.N. Bautin, E.A. Leontovich, "Methods and means for a qualitative investigation of dynamical systems on the plane" , Moscow (1976) (In Russian)

Comments

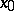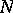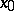The flow near a saddle node does not enjoy structural stability: If is a saddle node for (*), there is a neighbourhood of in 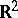such that for any 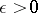there is a system having no equilibrium in 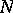, such that 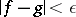and 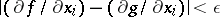, 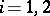. However, the saddle node bifurcation is robust and cannot be perturbed away ([a1]) (cf. also Rough system).

References

[a1]	J. Guckenheimer, P. Holmes, "Non-linear oscillations, dynamical systems, and bifurcations of vector fields" , Springer (1983)
[a2]	A.A. Andronov, E.A. Leontovich, I.I. Gordon, A.G. Maier, "Qualitative theory of second-order dynamic systems" , Wiley (1973) (Translated from Russian)