Quadrature formula of highest algebraic accuracy

A formula of the type

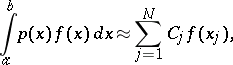(1)

where the weight function 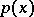is assumed to be non-negative on 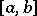, where the integrals

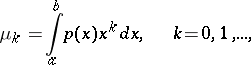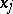exist and where, moreover, . The nodes of formula (1) are the roots of a polynomial of degree 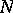orthogonal on 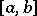with the weight function 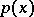, and the weights are defined by the condition that (1) be an interpolatory formula. A quadrature formula of this type has algebraic accuracy 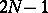, i.e. it is exact for all algebraic polynomials 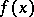of degree 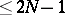and is not exact for 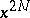; it is known as a quadrature formula of Gaussian type.

This concept can be generalized as follows. Consider the quadrature formula

(2)

with nodes, where the nodes 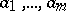are pre-assigned (fixed) while are so chosen that (2) be a quadrature formula of highest algebraic accuracy. Let

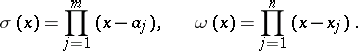Formula (2) is exact for all polynomials of degree 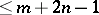if and only if it is an interpolatory quadrature formula and the polynomial is orthogonal on 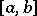with the weight function 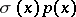to all polynomials of degree 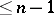. This reduces the question of the existence of a quadrature formula that is exact for all polynomials of degree 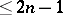to the problem of determining a polynomial 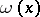of degree that is orthogonal on 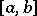with the weight function 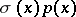, and to examining the properties of its roots. If the roots of 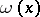are real, simple, lie in 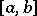, and none of them is one of the fixed nodes, the required quadrature formula exists. If, moreover,

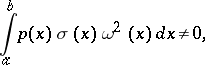then the algebraic accuracy of the formula is .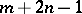

Under the above assumptions concerning the weight function 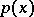, a polynomial 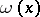of degree , orthogonal on 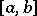with the weight function 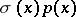, is defined uniquely (up to a non-zero constant factor) in the following special cases.

1) 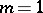, is arbitrary. The single fixed node is an end-point of the interval 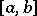, with the only condition that it be finite.

2) 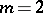, is arbitrary. The two fixed nodes are the end-points of the interval 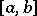, provided they are finite.

3) 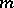is arbitrary, . The fixed nodes are the roots of a polynomial 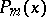that is orthogonal on 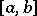with the weight function .

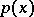In cases 1) and 2), the polynomial 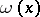is orthogonal relative to the weight function 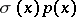, which is of fixed sign on 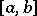, and therefore its roots are real, simple, lie inside 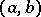, and are consequently distinct from 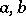. The quadrature formula (2) exists, its coefficients are positive and its algebraic accuracy is 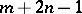. Quadrature formulas corresponding to the cases 1) and 2) are called Markov formulas.

In case 3), the weight function 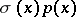changes sign on 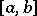and this complicates the inspection of the roots of 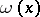. If and 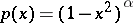, where 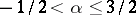, then the roots of 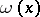lie inside 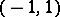and separate the roots of 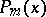: Between any two consecutive roots of 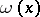there is exactly one root of 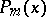(see [2]). With this weight function, the quadrature formula (2) exists and is exact for all polynomials of degree 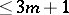; however, one cannot state that its algebraic accuracy is 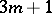. For and 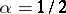the nodes and the coefficients of the quadrature formula can be specified explicitly (see [3]); the algebraic accuracy in the former case is increased to 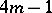, and in the second case to 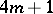. 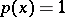For and the interval 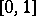, the nodes and the coefficients have been computed for the quadrature formula (2) (with fixed nodes of type 3)) for 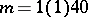(i.e. 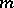varying from 1 to 40 with step 1) (see [4]); the algebraic accuracy is if 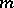is even and if 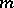is odd. 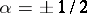A quadrature formula (2) with fixed nodes of type 3) also exists for the interval and the weight function for , and the nodes and the coefficients can be explicitly specified (see [3]).

References

[1]	V.I. Krylov, "Approximate calculation of integrals" , Macmillan (1962) (Translated from Russian)
[2]	G. Szegö, "Ueber gewisse orthogonale Polynome, die zu einer oszillierenden Belegungsfunktion gehören" Math. Ann. , 110 : 4 (1934) pp. 501–513
[3]	I.P Mysovskikh, "A special case of quadrature formulas containing pre-assigned nodes" Izv. Akad. Nauk BelorussSSR. Ser. Fiz.-Tekhn. Navuk , 4 (1964) pp. 125–127 (In Russian)
[4]	A.S. Kronrod, "Nodes and weights of quadrature formulas" , Consultants Bureau (1965) (Translated from Russian)

References

[a1]	P.J. Davis, P. Rabinowitz, "Methods of numerical integration" , Acad. Press (1975)