Poisson brackets
The differential expression
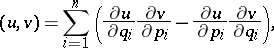 | (1) |
depending on two functions 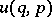 and
and 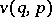 of
of 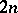 variables
variables 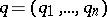 ,
, 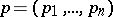 . The Poisson brackets, introduced by S. Poisson [1], are a particular case of the Jacobi brackets. The Poisson brackets are a bilinear form in the functions
. The Poisson brackets, introduced by S. Poisson [1], are a particular case of the Jacobi brackets. The Poisson brackets are a bilinear form in the functions  and
and  , such that
, such that
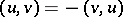 |
and the Jacobi identity
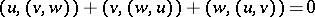 |
holds (see [2]).
The Poisson brackets are used in the theory of first-order partial differential equations and are a useful mathematical tool in analytical mechanics (see [3]–[5]). For example, if 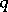 and
and 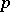 are canonical variables and a transformation
are canonical variables and a transformation
 | (2) |
is given, where 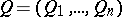 ,
, 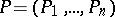 and the
and the 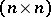 -matrices
-matrices
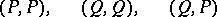 | (3) |
are constructed with entries 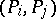 ,
, 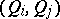 ,
, 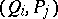 , respectively, then (2) is a canonical transformation if and only if the first two matrices in (3) are zero and the third is the unit matrix.
, respectively, then (2) is a canonical transformation if and only if the first two matrices in (3) are zero and the third is the unit matrix.
The Poisson brackets, computed for the case when  and
and  are replaced in (1) by some pair of coordinate functions in
are replaced in (1) by some pair of coordinate functions in 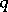 and
and 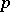 , are also called fundamental brackets.
, are also called fundamental brackets.
References
| [1] | S. Poisson, J. Ecole Polytechn. , 8 (1809) pp. 266–344 |
| [2] | C.G.J. Jacobi, "Nova methodus, aequationes differentiales partiales primi ordinis inter numurum variabilium quemcunque propositas integrandi" J. Reine Angew. Math. , 60 (1862) pp. 1–181 |
| [3] | E.T. Whittaker, "Analytical dynamics of particles and rigid bodies" , Dover, reprint (1944) |
| [4] | A.I. Lur'e, "Analytical mechanics" , Moscow (1961) (In Russian) |
| [5] | H. Goldstein, "Classical mechanics" , Addison-Wesley (1957) |
Comments
Other basic properties of Poisson brackets are invariance under canonical transformations and the fact that 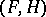 expresses the derivative of
expresses the derivative of 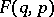 along trajectories, if
along trajectories, if 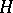 is the Hamiltonian, so that the corresponding Hamiltonian equations are
is the Hamiltonian, so that the corresponding Hamiltonian equations are 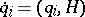 ,
,  , which for a "standard" Hamiltonian of the form
, which for a "standard" Hamiltonian of the form 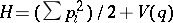 gives back
gives back  ,
,  . Therefore
. Therefore 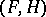 expresses a conservation law, i.e.
expresses a conservation law, i.e. 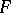 is a conserved quantity.
is a conserved quantity.
The Poisson brackets may be defined for functionals depending on a function 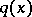 , as
, as
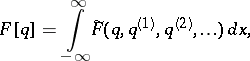 |
with 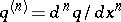 .
.
One has
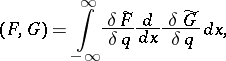 |
with  ,
, 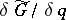 variational derivatives, i.e.
variational derivatives, i.e.
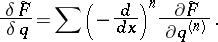 |
References
| [a1] | A.C. Newell, "Solitons in mathematical physics" , SIAM (1985) |
| [a2] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
| [a3] | R. Abraham, J.E. Marsden, "Foundations of mechanics" , Benjamin (1978) |
| [a4] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
Poisson brackets. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poisson_brackets&oldid=48215