Perturbation of a linear system

The mapping 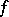in the system of ordinary differential equations

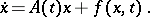(1)

A perturbation is usually assumed to be small in some sense, for example

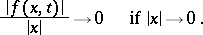(2)

The solution 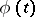of the perturbed system (1) and the solution of the linear system

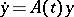(3)

with the same initial value 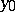at , are connected by the relation

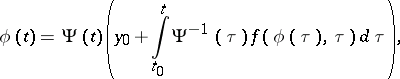known as the formula of variation of constants, where 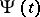is the fundamental matrix of the linear system (3).

It was shown by A.M. Lyapunov [1] that the trivial solution of the system (1) is asymptotically stable (cf. Asymptotically-stable solution) if relation (2) is valid uniformly in 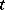, the matrix 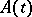is constant and all real parts of the eigen values of 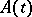are negative; if only one such real part is positive, the trivial solution is not stable.

The study of the periodic solution 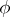of the system , describing an oscillating process, reduces in the general case by the transformation to the study of a perturbed linear system, the right-hand side of which is periodic in 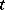[3].

References

[1]	A.M. Lyapunov, "Stability of motion" , Acad. Press (1966) (Translated from Russian)
[2]	B.F. Bylov, R.E. Vinograd, D.M. Grobman, V.V. Nemytskii, "The theory of Lyapunov exponents and its applications to problems of stability" , Moscow (1966) (In Russian)
[3]	L.S. Pontryagin, "Ordinary differential equations" , Addison-Wesley (1962) (Translated from Russian)

Comments

Results of this type are usually called Poincaré–Lyapunov theorems. There are several extensions, described, e.g., in [a1]. A recent tutorial text containing these matters is [a2].

References

[a1]	M. Roseau, "Vibrations non linéaires et théorie de la stabilité" , Springer (1966)
[a2]	F. Verhulst, "Nonlinear differential equations and dynamical systems" , Springer (1989)