Parametric representation method

A method in the theory of functions of a complex variable arising from the parametric representation of univalent functions and based to a large part on the Löwner equation and its generalizations (see [1]). K. Löwner himself used the method of parametric representation on the class 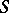of all regular univalent functions 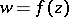, , 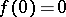, in the unit disc to estimate the coefficients of the expansions

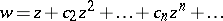and

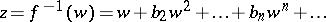(see Bieberbach conjecture). Later the method of parametric representation was applied systematically by G.M. Goluzin in the solution of problems of distortion, rotation, mutual growth, and other geometric characteristics of a mapping 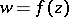connected with the values 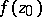and 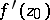for a fixed , .

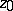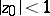The method of parametric representation is related to the theory of optimal processes. This link is based on the fact that all problems mentioned above can be stated analytically as extremal problems for a controlled system of ordinary differential equations obtained from Löwner's equations. The use of Pontryagin's maximum principle (see Pontryagin maximum principle) and the study of the properties of the Pontryagin function make it possible to study a number of new problems concerning the class 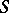and its subclasses right through to their complete solution, or to obtain results comparable (for example, in Bieberbach's problem) with results found by other methods (see [1]).

References

[1]	I.A. Aleksandrov, "Parametric representations in the theory of univalent functions" , Moscow (1976) (In Russian)

References

[a1]	P.L. Duren, "Univalent functions" , Springer (1983) pp. Sect. 10.11