Oscillating solution

A solution 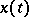of a differential equation

(*)

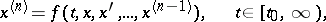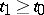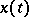with the property: There exists for any a point such that changes sign on passing through it. In many applied problems there arises the question of the existence of an oscillating solution or the question whether all the solutions of equation (*) oscillate. Many sufficient conditions are known under which equation (*) has an oscillating solution (see [1], [2], [3]). For example, any non-trivial solution of the equation 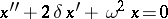with constant coefficients is oscillating if 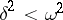; every non-trivial solution of the equation

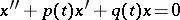with 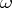-periodic coefficients is oscillating if

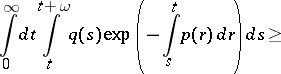and 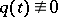on .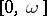

In a number of applications there arises the question of the existence of oscillating solutions (in the above sense) of a system of ordinary differential equations. For example, in control theory one studies the oscillation relative to a given hyperplane 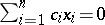of the solutions 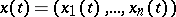of the system of equations 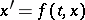, that is, the question whether the function 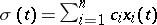oscillates. 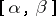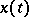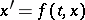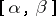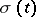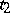-oscillating solutions are also studied; a bounded solution of the system is called -oscillating if is oscillating and for any there are points and 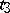such that 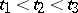, , 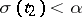, 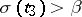where 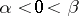. For the system 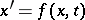there also exist other definitions of an oscillating solution.

References

[1]	P. Hartman, "Ordinary differential equations" , Birkhäuser (1982)
[2]	C.A. Swanson, "Comparison and oscillation theory of linear differential equations" , Acad. Press (1968)
[3]	I.T. Kiguradze, "Some singular boundary value problems for ordinary differential equations" , Tbilisi (1975) (In Russian)

References

[a1]	E.A. Coddington, N. Levinson, "Theory of ordinary differential equations" , McGraw-Hill (1955) pp. Chapts. 13–17