Orthogonality
A generalization of the concept of perpendicularity of vectors in a Euclidean space. The most natural concept of orthogonality is put forward in the theory of Hilbert spaces. Two elements  and
and  of a Hilbert space
of a Hilbert space  are said to be orthogonal
are said to be orthogonal  if their inner product is equal to zero (
if their inner product is equal to zero ( ). This concept of orthogonality in the particular case where
). This concept of orthogonality in the particular case where  is a Euclidean space coincides with the concept of perpendicularity of two vectors. In terms of this concept, in any Hilbert space Pythagoras' theorem holds: If an element
is a Euclidean space coincides with the concept of perpendicularity of two vectors. In terms of this concept, in any Hilbert space Pythagoras' theorem holds: If an element  is equal to a finite or countable sum of pairwise orthogonal elements
is equal to a finite or countable sum of pairwise orthogonal elements  (the countable sum
(the countable sum  is understood in the sense of convergence of the series in the metric of
is understood in the sense of convergence of the series in the metric of 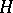 ), then
), then  (see Parseval equality).
(see Parseval equality).
A complete, countable, orthonormal system  in a separable Hilbert space is the analogue of a complete system of pairwise orthonormal vectors in a finite-dimensional Euclidean space: Any element
in a separable Hilbert space is the analogue of a complete system of pairwise orthonormal vectors in a finite-dimensional Euclidean space: Any element  can be uniquely represented as the sum
can be uniquely represented as the sum  , where
, where  is the orthogonal projection of the element
is the orthogonal projection of the element  onto the span of the vector
onto the span of the vector  .
.
E.g., in the function space  , if
, if  is a complete orthonormal system, then for every
is a complete orthonormal system, then for every  ,
,
 |
in the metric of the space  , where
, where
 |
When the  are bounded functions, the coefficients
are bounded functions, the coefficients  can be defined as above for any integrable function. In these cases the question of the convergence of a corresponding series in one sense or another is of interest (see Trigonometric system; Haar system). With respect to functions, therefore, the term "orthogonality" is used in a broader sense: Two functions
can be defined as above for any integrable function. In these cases the question of the convergence of a corresponding series in one sense or another is of interest (see Trigonometric system; Haar system). With respect to functions, therefore, the term "orthogonality" is used in a broader sense: Two functions  and
and 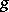 which are integrable on the segment
which are integrable on the segment  are orthogonal if
are orthogonal if
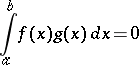 |
(for the integral to exist, it is usually required that  ,
,  ,
,  ,
,  , where
, where 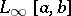 is the set of bounded measurable functions).
is the set of bounded measurable functions).
Definitions of orthogonality of elements of an arbitrary normed linear space also exist. One of them (see [4]) is as follows: An element  of a real normed space
of a real normed space  is considered orthogonal to the element
is considered orthogonal to the element  if
if  for all real
for all real  . In terms of this concept certain necessary and sufficient conditions have been established under which a scalar (inner) product of elements of
. In terms of this concept certain necessary and sufficient conditions have been established under which a scalar (inner) product of elements of 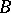 can be defined (see [5], [6]).
can be defined (see [5], [6]).
References
| [1] | L.V. Kantorovich, G.P. Akilov, "Functionalanalysis in normierten Räumen" , Akademie Verlag (1964) (Translated from Russian) |
| [2] | N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Wiley, reprint (1988) |
| [3] | S. Kaczmarz, H. Steinhaus, "Theorie der Orthogonalreihen" , Chelsea, reprint (1951) |
| [4] | G. Birkhoff, "Orthogonality in linear metric spaces" Duke Math. J. , 1 (1935) pp. 169–172 |
| [5] | R. James, "Orthogonality and linear functionals in normed linear spaces" Trans. Amer. Math. Soc. , 61 (1947) pp. 265–292 |
| [6] | R. James, "Inner products in normed linear spaces" Bull. Amer. Math. Soc. , 53 (1947) pp. 559–566 |
Comments
References
| [a1] | D. Amir, "Characterizations of inner product spaces" , Birkhäuser (1986) |
| [a2] | N.I. [N.I. Akhiezer] Achieser, I.M. [I.M. Glaz'man] Glasman, "Theorie der linearen Operatoren im Hilbert Raum" , Akademie Verlag (1958) (Translated from Russian) |
| [a3] | V.I. Istrăţescu, "Inner product structures" , Reidel (1987) |
Orthogonality. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Orthogonality&oldid=48081