Normal p-complement
of a finite group 
A normal subgroup 
such that 
and 
, where 
is a Sylow 
-subgroup of 
(see Sylow subgroup). A group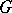 
has a normal 
-complement if some Sylow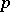 
-subgroup 
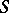of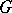 
lies in the centre of its normalizer (cf. Normalizer of a subset) (Burnside's theorem). A necessary and sufficient condition for the existence of a normal 
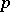-complement in a group 
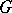is given by Frobenius' theorem: A group 
has a normal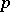 
-complement if and only either for any non-trivial 
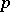-subgroup 
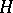of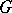 
the quotient group 
is a 
-group (where 
is the normalizer and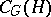 
the centralizer of 
in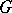 
) or if for every non-trivial 
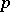-subgroup 
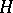of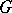 
the subgroup 
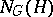has a normal 
-complement.
References
| [1] | D. Gorenstein, "Finite groups" , Harper & Row (1968) |
Comments
Let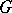 
be a group of order 
and let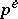 
be the highest power of a prime number 
dividing 
. A subgroup of 
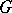of index 
(and hence of order 
) is called a 
-complement in 
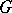. A normal 
-complement is a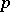 
-complement that is normal. A finite group is solvable if and only if it has a 
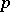-complement for every prime number 
dividing its order. Cf. [a1], [a2] for more details; cf. also Hall subgroup.
References
| [a1] | M. Hall jr., "The theory of groups" , Macmillan (1959) pp. Sect. 9.3 |
| [a2] | B. Huppert, "Endliche Gruppen" , 1 , Springer (1967) pp. Sect. VI.1 |
Normal p-complement. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Normal_p-complement&oldid=48016