Normal family
of analytic functions in a domain
A family 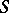 of single-valued analytic functions
of single-valued analytic functions 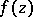 of complex variables
of complex variables 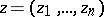 in a domain
in a domain 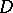 in the space
in the space 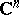 ,
, 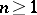 , such that from any sequence of functions in
, such that from any sequence of functions in 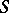 one can extract a subsequence
one can extract a subsequence 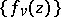 that converges uniformly on compact subsets in
that converges uniformly on compact subsets in 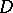 to an analytic function or to infinity. Uniform convergence to infinity on compact subsets means, by definition, that for any compact set
to an analytic function or to infinity. Uniform convergence to infinity on compact subsets means, by definition, that for any compact set 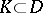 and any
and any 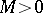 one can find an
one can find an 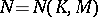 such that
such that 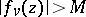 for all
for all 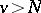 ,
, 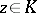 .
.
A family 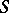 is called a normal family at a point
is called a normal family at a point 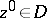 if
if  is normal in some ball with centre at
is normal in some ball with centre at 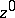 . A family
. A family 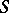 is normal in
is normal in 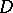 if and only if it is normal at every point
if and only if it is normal at every point 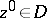 . Every compact family of holomorphic functions is normal; the converse conclusion is false (see Compactness principle). If a family
. Every compact family of holomorphic functions is normal; the converse conclusion is false (see Compactness principle). If a family 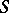 of holomorphic functions in a domain
of holomorphic functions in a domain 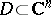 has the property that all functions
has the property that all functions 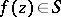 omit two fixed values, then
omit two fixed values, then 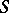 is normal in
is normal in 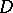 (Montel's theorem). This criterion of normality considerably simplifies the investigation of analytic functions in a neighbourhood of an essential singular point (see also Picard theorem).
(Montel's theorem). This criterion of normality considerably simplifies the investigation of analytic functions in a neighbourhood of an essential singular point (see also Picard theorem).
A normal family of meromorphic functions in a domain 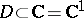 is defined similarly: A family
is defined similarly: A family 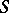 of meromorphic functions in
of meromorphic functions in 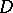 is normal if from every sequence of functions in
is normal if from every sequence of functions in 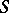 one can extract a subsequence
one can extract a subsequence 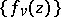 that converges uniformly on compact subsets in
that converges uniformly on compact subsets in 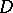 to a meromorphic function or to infinity. By definition,
to a meromorphic function or to infinity. By definition,  converges uniformly on compact subsets in
converges uniformly on compact subsets in  to
to 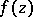 (the case
(the case 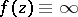 is excluded) if for any compact set
is excluded) if for any compact set 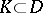 and any
and any 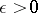 there is an
there is an 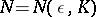 and a disc
and a disc 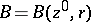 of radius
of radius 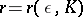 with centre at some point
with centre at some point 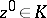 such that for
such that for 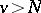 ,
,
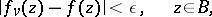 |
when 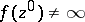 , or
, or
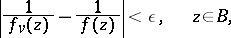 |
when 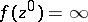 . If a family
. If a family  of meromorphic functions in a domain
of meromorphic functions in a domain 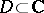 has the property that all functions
has the property that all functions 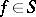 omit three fixed values, then
omit three fixed values, then  is normal (Montel's theorem). A family
is normal (Montel's theorem). A family 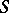 of meromorphic functions is normal in a domain
of meromorphic functions is normal in a domain  if and only if
if and only if
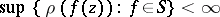 |
on every compact set  , where
, where
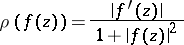 |
is the so-called spherical derivative of  .
.
From the 1930s onwards great value was attached to the study of boundary properties of analytic functions (see also Cluster set, [3], [4]). A meromorphic function 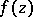 in a simply-connected domain
in a simply-connected domain 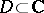 is said to be a normal function in the domain
is said to be a normal function in the domain 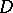 if the family
if the family 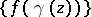 is normal in
is normal in 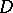 , where
, where 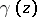 ranges over the family of all conformal automorphisms of
ranges over the family of all conformal automorphisms of 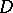 . A function
. A function  is called normal in a multiply-connected domain
is called normal in a multiply-connected domain  if it is normal on the universal covering surface of
if it is normal on the universal covering surface of 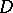 . If a meromorphic function
. If a meromorphic function 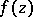 in
in 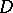 omits three values, then
omits three values, then 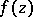 is normal. For
is normal. For  ,
, 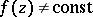 , to be normal in the unit disc
, to be normal in the unit disc 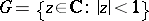 it is necessary and sufficient that
it is necessary and sufficient that
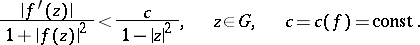 |
For a normal meromorphic function 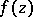 in the unit disc
in the unit disc 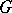 the existence of an asymptotic value
the existence of an asymptotic value  at a boundary point
at a boundary point 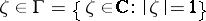 implies that
implies that 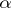 is a non-tangential boundary value (cf. Angular boundary value) of
is a non-tangential boundary value (cf. Angular boundary value) of 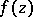 at
at 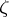 . However, a meromorphic normal function in
. However, a meromorphic normal function in 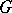 need not have asymptotic values at all. On the other hand, if
need not have asymptotic values at all. On the other hand, if 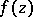 is a holomorphic normal function in
is a holomorphic normal function in 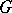 , then non-tangential boundary values exist even on a set of points of the unit circle
, then non-tangential boundary values exist even on a set of points of the unit circle  that is dense in
that is dense in 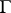 .
.
References
| [1] | P. Montel, "Leçons sur les familles normales de fonctions analytiques et leurs applications" , Gauthier-Villars (1927) |
| [2] | A.I. Markushevich, "Theory of functions of a complex variable" , 2 , Chelsea (1977) (Translated from Russian) |
| [3] | E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966) pp. Chapt. 1;6 |
| [4] | A. Lohwater, "The boundary behaviour of analytic functions" Itogi Nauk. i Tekhn. Mat. Anal. , 10 (1973) pp. 99–259 (In Russian) |
Comments
Let  ,
,  be domains. A family
be domains. A family  of analytic mappings from
of analytic mappings from 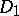 to
to  is called normal if from any sequence of mappings in
is called normal if from any sequence of mappings in 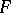 one can either extract a subsequence
one can either extract a subsequence 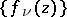 that is uniformly convergent on compact subsets in
that is uniformly convergent on compact subsets in 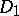 to an analytic mapping from
to an analytic mapping from 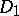 to
to 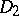 , or a subsequence
, or a subsequence  with the property that for every compact sets
with the property that for every compact sets 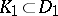 ,
,  there is an
there is an 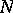 such that
such that 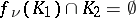 for
for 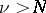 , see [a1].
, see [a1].
References
| [a1] | S.G. Krantz, "Function theory of several complex variables" , Wiley (1982) |
| [a2] | O. Lehto, K.I. Virtanen, "Boundary behaviour and normal meromorphic functions" Acta Math. , 97 (1957) pp. 47–65 |
Normal family. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Normal_family&oldid=48012