Modulus of an automorphism

A positive real number associated to an automorphism of a locally compact group. Let be such a group and let be an automorphism of , regarded as a topological group. Then the modulus of is defined by

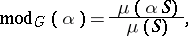where is left-invariant Haar measure on and is any compact subset of with positive measure (indeed, 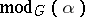does not depend on 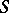). If is compact or discrete, then , since for a compact group one can put , and for a discrete group one can take 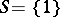, where 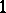is the identity element of .

If and 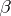are two automorphism of 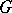, then

If 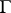is a topological group which acts continuously on by automorphisms, then the associated homomorphism 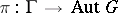defines a continuous homomorphism 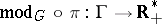, where is the multiplicative group of positive real numbers. In particular, if and , then 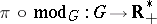is a continuous homomorphism. This homomorphism is trivial if and only if the left-invariant Haar measure on 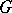is simultaneously right invariant. Groups satisfying the latter condition are called unimodular.

If is a locally compact skew-field, then each non-zero element defines an automorphism of the additive group of via multiplication by . The function 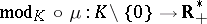is used in the study of the structure of locally compact skew-fields.

References

[1]	N. Bourbaki, "Elements of mathematics. Integration" , Addison-Wesley (1975) pp. Chapt.6;7;8 (Translated from French)
[2]	A. Weil, "l'Intégration dans les groupes topologiques et ses applications" , Hermann (1940)
[3]	A. Weil, "Basic number theory" , Springer (1974)