Micro-bundle
A mapping  which is a retraction (that is, there is a
which is a retraction (that is, there is a 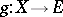 for which
for which 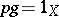 ) and which is locally trivial in the sense that for each
) and which is locally trivial in the sense that for each 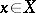 there is a neighbourhood
there is a neighbourhood 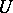 of
of 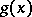 in
in  which can be represented as a direct product
which can be represented as a direct product 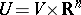 , with
, with 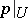 the projection onto
the projection onto 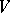 . If for each such neighbourhood
. If for each such neighbourhood 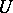 there is fixed a piecewise-linear structure in each fibre
there is fixed a piecewise-linear structure in each fibre 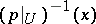 , if, moreover, the projection of
, if, moreover, the projection of  on
on 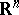 is piecewise linear and for two neighbourhoods
is piecewise linear and for two neighbourhoods 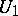 and
and 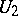 and any
and any 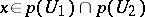 the structures on
the structures on 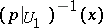 and
and  coincide in a neighbourhood of
coincide in a neighbourhood of 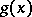 , then the micro-bundle is called piecewise linear. Other structures may be introduced similarly.
, then the micro-bundle is called piecewise linear. Other structures may be introduced similarly.
The notion of a micro-bundle was introduced in order to define an analogue of the tangent bundle for a topological or piecewise-linear manifold 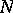 . Namely, here
. Namely, here 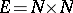 ,
,  and
and 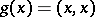 . Each topological micro-bundle is equivalent to a unique locally trivial bundle with fibres
. Each topological micro-bundle is equivalent to a unique locally trivial bundle with fibres 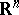 of corresponding dimension, that is, there is a homeomorphism
of corresponding dimension, that is, there is a homeomorphism 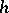 of some neighbourhood
of some neighbourhood 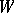 of
of 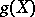 in
in 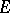 into a neighbourhood
into a neighbourhood 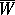 of the zero section of some bundle
of the zero section of some bundle  with fibre
with fibre 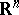 . This fact is also true for piecewise-linear micro-bundles. Despite the fact that, because of this theorem, the notion of a micro-bundle has lost its theoretical interest, it is still used in concrete problems.
. This fact is also true for piecewise-linear micro-bundles. Despite the fact that, because of this theorem, the notion of a micro-bundle has lost its theoretical interest, it is still used in concrete problems.
Comments
References
| [a1] | J. Milnor, "Microbundles, Part I" Topology , 3, Suppl. 1 (1964) pp. 53–80 |
| [a2] | R.C. Kirby, L.C. Siebenmann, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977) |
Micro-bundle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Micro-bundle&oldid=47835