Metric connection
A linear connection in a vector bundle 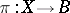 , equipped with a bilinear form in the fibres, for which parallel displacement along an arbitrary piecewise-smooth curve in
, equipped with a bilinear form in the fibres, for which parallel displacement along an arbitrary piecewise-smooth curve in  preserves the form, that is, the scalar product of two vectors remains constant under parallel displacement. If the bilinear form is given by its components
preserves the form, that is, the scalar product of two vectors remains constant under parallel displacement. If the bilinear form is given by its components  and the linear connection by a matrix
and the linear connection by a matrix 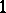 -form
-form  , then this connection is metric if
, then this connection is metric if
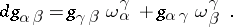 |
In the case of a non-degenerate symmetric bilinear form, i.e. 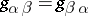 and
and  , the metric connection is called a Euclidean connection. In the case of a non-degenerate skew-symmetric bilinear form, the metric connection is called a symplectic connection in the vector bundle.
, the metric connection is called a Euclidean connection. In the case of a non-degenerate skew-symmetric bilinear form, the metric connection is called a symplectic connection in the vector bundle.
Under projectivization of a vector bundle, when the symmetric bilinear form generates some projective metric in each fibre (as in a projective space), the role of the metric connection is played by the projective-metric connection.
Comments
In the case of a positive-definite bilinear form, the metric connection is also called a Riemannian connection.
References
| [a1] | W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German) |
| [a2] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1969) |
Metric connection. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Metric_connection&oldid=47827