Maurer-Cartan form

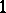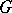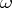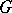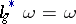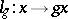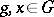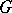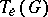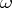A left-invariant -form on a Lie group , i.e. a differential form of degree 1 on satisfying the condition for any left translation , . The Maurer–Cartan forms on are in one-to-one correspondence with the linear forms on the tangent space at the point ; specifically, the mapping which sends each Maurer–Cartan form to its value 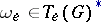is an isomorphism of the space of Maurer–Cartan forms onto 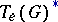. The differential of a Maurer–Cartan form is a left-invariant -form on 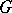, defined by the formula

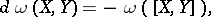(1)

where 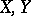are arbitrary left-invariant vector fields on 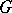. Suppose that 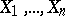is a basis in 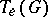and let , , 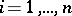be Maurer–Cartan forms such that

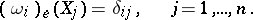Then

(2)

where 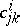are the structure constants of the Lie algebra 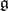of 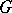consisting of the left-invariant vector fields on 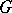, with respect to the basis determined by

The equalities (2) (or (1)) are called the Maurer–Cartan equations. They were first obtained (in a different, yet equivalent form) by L. Maurer [1]. The forms were introduced by E. Cartan in 1904 (see [2]).

Let be the canonical coordinates in a neighbourhood of the point determined by the basis 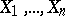. Then the forms 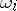are written in the form

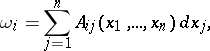in which the matrix

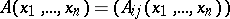is calculated by the formula

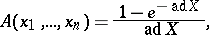where 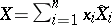and 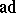is the adjoint representation of the Lie algebra .

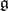Furthermore, let 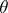be the 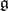-valued 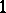-form on 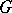which assigns to each tangent vector to 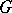the unique left-invariant vector field containing this vector ( 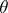is called the canonical left differential form). Then

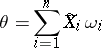and

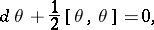which is yet another way of writing the Maurer–Cartan equations.

References

[1]	L. Maurer, Sitzungsber. Bayer. Akad. Wiss. Math. Phys. Kl. (München) , 18 (1879) pp. 103–150
[2]	E. Cartan, "Sur la structure des groupes infinis de transformations" Ann. Ecole Norm. , 21 (1904) pp. 153–206
[3]	C. Chevalley, "Theory of Lie groups" , 1 , Princeton Univ. Press (1946)
[4]	N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French)
[5]	S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978)