Marginal distribution
The distribution of a random variable, or set of random variables, obtained by considering a component, or subset of components, of a larger random vector (see Multi-dimensional distribution) with a given distribution. Thus the marginal distribution is the projection of the distribution of the random vector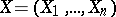 
onto an axis 
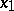or subspace defined by variables 
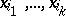, and is completely determined by the distribution of the original vector. For example, if 
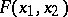is the distribution function of 
in 
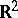, then the distribution function of 
is equal to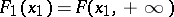 
; if the two-dimensional distribution is absolutely continuous and if 
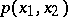is its density, then the density of the marginal distribution of 
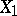is
 |
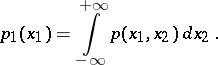The marginal distribution is calculated similarly for any component or set of components of the vector 
for any 
. If the distribution of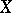 
is normal, then all marginal distributions are also normal. When 
are mutually independent, then the distribution of 
is uniquely determined by the marginal distributions of the components 
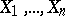of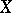 
:
 |
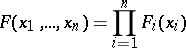and
 |
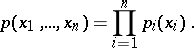The marginal distribution with respect to a probability distribution given on a product of spaces more general than real lines is defined similarly.
References
| [1] | M. Loève, "Probability theory" , Springer (1977) |
| [2] | H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946) |
Marginal distribution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Marginal_distribution&oldid=47762