Locally finite family
From Encyclopedia of Mathematics
of sets in a topological space
A family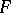 
of sets such that every point of the space has a neighbourhood that intersects only finitely many elements of 
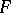. Locally finite families of open sets and locally finite open coverings are important. Thus, a regular space is metrizable if and only if has a base that splits into countably many locally finite families. Any open covering of a metric space can be refined to a locally finite open covering. Spaces that have this property are called paracompact (cf. Paracompact space).
Comments
See also Locally finite covering.
How to Cite This Entry:
Locally finite family. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Locally_finite_family&oldid=47695
Locally finite family. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Locally_finite_family&oldid=47695
This article was adapted from an original article by A.V. Arkhangel'skii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article