The integral transform
where 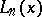 is the Laguerre polynomial (cf. Laguerre polynomials) of degree
is the Laguerre polynomial (cf. Laguerre polynomials) of degree  . The inversion formula is
. The inversion formula is
if the series converges. If 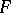 is continuous,
is continuous,  is piecewise continuous on
is piecewise continuous on  and
and 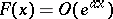 ,
, 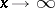 ,
, 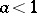 , then
, then
If 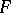 and
and  are continuous,
are continuous, 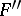 is piecewise continuous on
is piecewise continuous on  and
and  ,
, 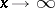 ,
,  , then
, then
If 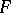 is piecewise continuous on
is piecewise continuous on  and
and  ,
, 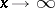 ,
, 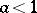 , then for
, then for
and for  ,
,
Suppose that 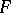 and
and 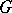 are piecewise continuous on
are piecewise continuous on  and that
and that
Then
The generalized Laguerre transform is
where 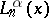 is the generalized Laguerre polynomial (see [4]).
is the generalized Laguerre polynomial (see [4]).
References
| [1] | A.G. Zemanian, "Generalized integral transformations" , Interscience (1968) |
| [2] | J. McCully, "The Laguerre transform" SIAM Rev. , 2 : 3 (1960) pp. 185–191 |
| [3] | L. Debnath, "On Laguerre transform" Bull. Calcutta Math. Soc. , 52 : 2 (1960) pp. 69–77 |
| [4] | Yu.A. Brychkov, A.P. Prudnikov, "Operational calculus" Progress in Math. , 1 (1968) pp. 1–74 Itogi Nauk. Mat. Anal. 1966 (1967) pp. 7–82 |
How to Cite This Entry:
Laguerre transform. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laguerre_transform&oldid=47567
This article was adapted from an original article by Yu.A. BrychkovA.P. Prudnikov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article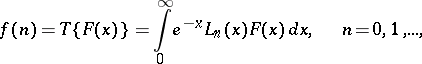
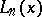 is the Laguerre polynomial (cf. Laguerre polynomials) of degree
is the Laguerre polynomial (cf. Laguerre polynomials) of degree  . The inversion formula is
. The inversion formula is
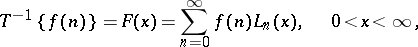
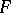 is continuous,
is continuous,  is piecewise continuous on
is piecewise continuous on  and
and 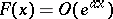 ,
, 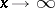 ,
, 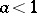 , then
, then
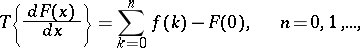

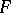 and
and  are continuous,
are continuous, 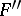 is piecewise continuous on
is piecewise continuous on  and
and  ,
, 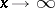 ,
,  , then
, then
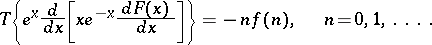
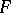 is piecewise continuous on
is piecewise continuous on  and
and  ,
, 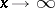 ,
, 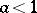 , then for
, then for
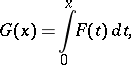
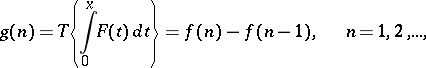
 ,
,
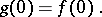
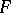 and
and 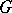 are piecewise continuous on
are piecewise continuous on  and that
and that
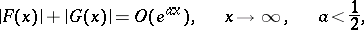

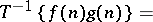
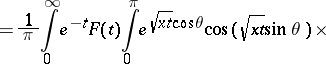



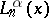 is the generalized Laguerre polynomial (see [4]).
is the generalized Laguerre polynomial (see [4]).