Lagrange bracket
Lagrange brackets, with respect to variables  and
and 
A sum of the form
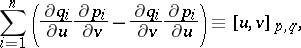 | (*) |
where  and
and  are certain functions of
are certain functions of  and
and  .
.
If  and
and  are canonical variables and
are canonical variables and  ,
, 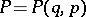 are canonical transformations, then the Lagrange bracket is an invariant of this transformation:
are canonical transformations, then the Lagrange bracket is an invariant of this transformation:
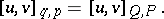 |
For this reason the indices  on the right-hand side of (*) are often omitted. The Lagrange bracket is said to be fundamental when the variables
on the right-hand side of (*) are often omitted. The Lagrange bracket is said to be fundamental when the variables  and
and  coincide with some pair of the
coincide with some pair of the 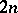 variables
variables  . From them one can form three matrices:
. From them one can form three matrices:
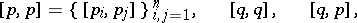 |
the first two of which are the zero, and the last one is the unit matrix. There is a definite connection between Lagrange brackets and Poisson brackets. Namely, if the functions 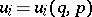 ,
,  , induce a diffeomorphism
, induce a diffeomorphism  , then the matrices formed from the elements
, then the matrices formed from the elements 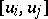 and
and  are inverse to each other.
are inverse to each other.
References
| [1] | J.L. Lagrange, "Oeuvres" , 6 , Gauthier-Villars (1873) |
| [2] | E.T. Whittaker, "Analytical dynamics of particles and rigid bodies" , Dover, reprint (1944) |
| [3] | A.I. Lur'e, "Analytical mechanics" , Moscow (1961) (In Russian) |
| [4] | H. Goldstein, "Classical mechanics" , Addison-Wesley (1957) |
Comments
If  denotes the mapping:
denotes the mapping:  , then the Lagrange bracket is equal to the product of the vectors
, then the Lagrange bracket is equal to the product of the vectors  and
and  with respect to the canonical symplectic form (cf. Symplectic manifold) on the phase space. More generally, if
with respect to the canonical symplectic form (cf. Symplectic manifold) on the phase space. More generally, if  is a symplectic form on a smooth manifold
is a symplectic form on a smooth manifold 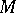 and
and  is a smooth mapping from a surface
is a smooth mapping from a surface  to
to  , then
, then 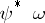 is an area form on
is an area form on 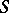 . If
. If  is a standard area form on
is a standard area form on  , then the function
, then the function  on
on  could be called the Lagrange brackets of
could be called the Lagrange brackets of 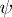 . See [a1], Chapt. 3.
. See [a1], Chapt. 3.
References
| [a1] | R. Abraham, J.E. Marsden, "Foundations of mechanics" , Benjamin/Cummings (1978) |
| [a2] | F.R. [F.R. Gantmakher] Gantmacher, "Lectures in analytical mechanics" , MIR (1975) (Translated from Russian) |
Lagrange bracket. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lagrange_bracket&oldid=47553