Krull ring

Krull domain

A commutative integral domain with the following property: There exists a family of discrete valuations on the field of fractions (cf. Fractions, ring of) of such that: a) for any and all , except possibly a finite number of them, ; and b) for any , if and only if for all 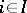. Under these conditions, is said to be an essential valuation.

Krull rings were first studied by W. Krull [1], who called them rings of finite discrete principal order. They are the most natural class of rings in which there is a divisor theory (see also Divisorial ideal; Divisor class group). The ordered group of divisors of a Krull ring is canonically isomorphic to the ordered group . The essential valuations of a Krull ring may be identified with the set of prime ideals of height 1. A Krull ring is completely integrally closed. Any integrally-closed Noetherian integral domain, in particular a Dedekind ring, is a Krull ring. The ring 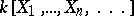of polynomials in infinitely many variables is an example of a Krull ring which is not Noetherian. In general, any factorial ring is a Krull ring. A Krull ring is a factorial ring if and only if every prime ideal of height 1 is principal.

The class of Krull rings is closed under localization, passage to the ring of polynomials or formal power series, and also under integral closure in a finite extension of the field of fractions .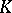

References

[1]	W. Krull, "Allgemeine Bewertungstheorie" J. Reine Angew. Math. , 167 (1931) pp. 160–196
[2]	O. Zariski, P. Samuel, "Commutative algebra" , 2 , Springer (1975)
[3]	N. Bourbaki, "Elements of mathematics. Commutative algebra" , Addison-Wesley (1972) (Translated from French)

References

[a1]	R.M. Fossum, "The divisor class group of a Krull domain" , Springer (1973)