Kotel'nikov interpretation

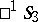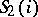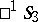An interpretation of the manifold of straight lines in the three-dimensional Lobachevskii space on the complex plane (or on ). With every straight line in one associates its Plücker coordinates, which are defined in this case up to sign. Using these line coordinates one establishes a correspondence between the straight lines and their polars in 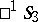, and also defines vectors of lines and their polars. One of two mutually-polar lines is represented by a vector of unit length, and the other by a vector of imaginary unit length. The manifold of pairs of mutually-polar straight lines of 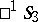is represented by the plane 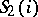with radius of curvature 1 or 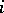, and this correspondence is continuous. Isotropic straight lines in 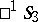are represented by points of the absolute in 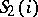. The connected group of motions of the space 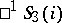is isomorphic to the group of motions of the plane .

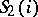The Kotel'nikov interpretation is sometimes understood in a broader sense, as the interpretation of manifolds of straight lines in three-dimensional spaces as complex or other two-dimensional planes (see Fubini model).

Kotel'nikov interpretations were first proposed by A.P. Kotel'nikov (see [1]) and independently by E. Study (see [2]).

References

[1]	A.P. Kotel'nikov, "Projective theory of vectors" , Kazan' (1899) (In Russian)
[2]	E. Study, "Geometrie der Dynamen" , Teubner (1903)
[3]	B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian)

Comments

One also encounters Kotel'nikov model instead of interpretation.

References

[a1]	B.A. [B.A. Rozenfel'd] Rosenfel'd, "A history of non-euclidean geometry" , Springer (1988) (Translated from Russian)