Kirchhoff formula

Kirchhoff integral

The formula

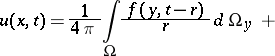(1)

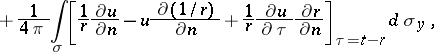expressing the value 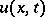of the solution of the inhomogeneous wave equation

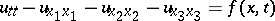(2)

at the point 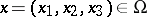at the instant of time 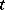in terms of the retarded volume potential

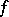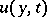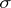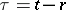with density , and in terms of the values of the function and its first-order derivatives on the boundary of the domain at the instant of time . Here is a bounded domain in the three-dimensional Euclidean space with a piecewise-smooth boundary 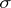, is the outward normal to 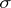and 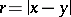is the distance between and .

Let

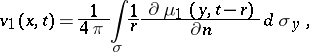where

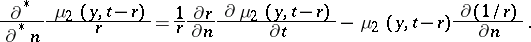The integrals 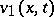and are called the retarded potentials of the single and the double layer.

The Kirchhoff formula (1) means that any twice continuously-differentiable solution 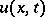of equation (2) can be expressed as the sum of the retarded potentials of a single layer, a double layer and a volume potential:

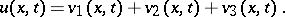In the case when and 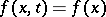do not depend on 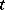, the Kirchhoff formula takes the form

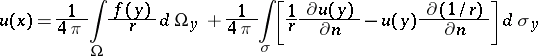and gives a solution of the Poisson equation .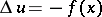

The Kirchhoff formula is widely applied in the solution of a whole series of problems. For example, if is the ball 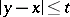of radius 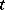and centre , then formula (1) is transformed into the relation

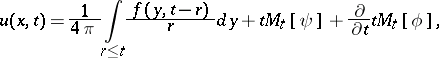(3)

where

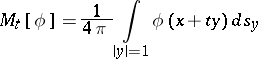is the average value of 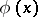over the surface of the sphere ,

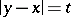(4)

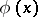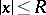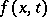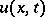If and are given functions in the ball , with continuous partial derivatives of orders three and two, respectively, and is a twice continuously-differentiable function for , , then the function defined by (3) is a regular solution of the Cauchy problem (4) for equation (2) when and .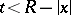

Formula (3) is also called Kirchhoff's formula.

The Kirchhoff formula in the form

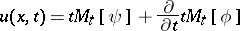for the wave equation

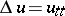(5)

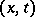is remarkable in that the Huygens principle does follow from it: The solution (wave) of (5) at the point of the space of independent variables 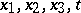is completely determined by the values of , 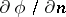and 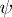on the sphere 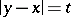with centre at and radius .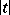

Consider the following equation of normal hyperbolic type:

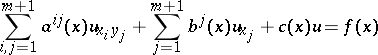(6)

with sufficiently-smooth coefficients 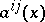, , 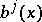, 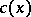and right-hand side 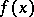in some 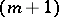-dimensional domain , that is, a form

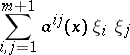that at any point 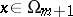can be reduced by means of a non-singular linear transformation to the form

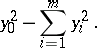The Kirchhoff formula generalizes to equation (6) in the case when the number 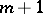of independent variables 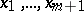is even [4]. Here the essential point is the construction of the function 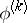that generalizes the Newton potential 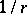to the case of equation (6). For the special case of equation (6),

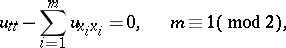(7)

the generalized Kirchhoff formula is

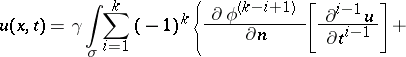(8)

where 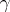is a positive number, 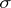is the piecewise-smooth boundary of an -dimensional bounded domain 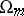containing the point 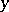in its interior, and is the outward normal to 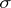. Further,

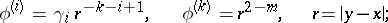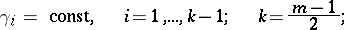and 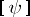denotes the retarded value of :

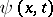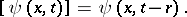Formula (8) for equation (6) is sometimes called the Kirchhoff–Sobolev formula.

References

[1]	A.V. Bitsadze, "Equations of mathematical physics" , MIR (1980) (Translated from Russian)
[2]	V.S. Vladimirov, "Equations of mathematical physics" , MIR (1984) (Translated from Russian)
[3]	H. Bateman, "Partial differential equations of mathematical physics" , Dover (1944)
[4]	M. Mathisson, "Eine neue Lösungsmethode für Differentialgleichungen von normalem hyperbolischem Typus" Math. Ann. , 107 (1932) pp. 400–419
[5]	M. Mathisson, "Le problème de M. Hadamard rélatifs à la diffusion des ondes" Acta Math. , 71 : 3–4 (1939) pp. 249–282
[6]	S.G. Mikhlin, "Linear partial differential equations" , Moscow (1977) (In Russian)
[7]	S.L. Sobolev, "Sur une généralisation de la formule de Kirchhoff" Dokl. Akad. Nauk SSSR , 1 : 6 (1933) pp. 256–262
[8]	S.L. Sobolev, "Applications of functional analysis in mathematical physics" , Amer. Math. Soc. (1963) (Translated from Russian)
[9]	V.I. Smirnov, "A course of higher mathematics" , 4 , Addison-Wesley (1964) (Translated from Russian)
[10]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)

References

[a1]	B.B. Baker, E.T. Copson, "The mathematical theory of Huygens's principle" , Clarendon Press (1950)
[a2]	L. Schwartz, "Théorie des distributions" , 2 , Hermann (1951)
[a3]	G.R. Kirchhoff, "Vorlesungen über mathematischen Physik" Ann. der Physik , 18 (1883)