Killing vector
more precisely, Killing vector field or infinitesimal motion
The field of velocities of a (local) one-parameter group of motions on a Riemannian manifold  . More precisely, a vector field
. More precisely, a vector field 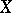 on
on  is called a Killing vector field if it satisfies the Killing equation
is called a Killing vector field if it satisfies the Killing equation
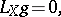 | (*) |
where  is the Lie derivative along
is the Lie derivative along  and
and  is the Riemannian metric of
is the Riemannian metric of 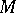 . These fields were first systematically studied by W. Killing [1], who also introduced equation (*) for them. In a complete Riemannian manifold any Killing vector field is complete, that is, it is the field of velocities of a one-parameter group of motions. The set
. These fields were first systematically studied by W. Killing [1], who also introduced equation (*) for them. In a complete Riemannian manifold any Killing vector field is complete, that is, it is the field of velocities of a one-parameter group of motions. The set 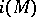 of all Killing vector fields on
of all Killing vector fields on 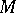 forms a Lie algebra of dimension not exceeding
forms a Lie algebra of dimension not exceeding 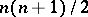 , where
, where  , and this dimension is equal to
, and this dimension is equal to 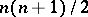 only for spaces of constant curvature. The set of all complete Killing vector fields forms a subalgebra of
only for spaces of constant curvature. The set of all complete Killing vector fields forms a subalgebra of 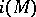 , which is the Lie algebra of the group of motions of
, which is the Lie algebra of the group of motions of 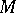 . The Lie derivative along the direction of a Killing vector field annihilates not only the metric
. The Lie derivative along the direction of a Killing vector field annihilates not only the metric 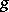 but also all fields that are canonically constructed in terms of the metric, for example, the Riemann curvature tensor, the Ricci operator, etc. This enables one to establish a connection between the properties of Killing vector fields and the curvature tensor. For example, at a point where all eigen values of the Ricci operator are distinct, a Killing vector field cannot vanish.
but also all fields that are canonically constructed in terms of the metric, for example, the Riemann curvature tensor, the Ricci operator, etc. This enables one to establish a connection between the properties of Killing vector fields and the curvature tensor. For example, at a point where all eigen values of the Ricci operator are distinct, a Killing vector field cannot vanish.
A Killing vector field 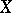 , regarded as a function
, regarded as a function
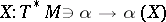 |
on the cotangent bundle 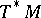 , is a first integral of the (Hamilton) geodesic flow on
, is a first integral of the (Hamilton) geodesic flow on 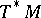 determined by the Riemannian metric. Analogously, a field
determined by the Riemannian metric. Analogously, a field 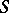 of contravariant symmetric tensors on
of contravariant symmetric tensors on 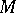 is called a Killing tensor field if the function
is called a Killing tensor field if the function
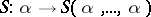 |
on 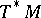 (polynomial on the fibres) corresponding to it is a first integral of the geodesic flow. The equation determining a Killing tensor field is also called the Killing equation. The set of all Killing tensor fields, regarded as functions on
(polynomial on the fibres) corresponding to it is a first integral of the geodesic flow. The equation determining a Killing tensor field is also called the Killing equation. The set of all Killing tensor fields, regarded as functions on  , forms a (generally infinite-dimensional) Lie algebra with respect to the Poisson brackets defined by the standard symplectic structure on
, forms a (generally infinite-dimensional) Lie algebra with respect to the Poisson brackets defined by the standard symplectic structure on 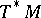 .
.
More generally, let  be a geometric object of order
be a geometric object of order 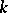 on the manifold
on the manifold 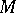 , that is, a
, that is, a 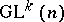 -equivariant mapping of the manifold of
-equivariant mapping of the manifold of  -frames on
-frames on  into the space
into the space 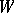 on which the group
on which the group 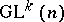 of
of 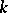 -jets of diffeomorphisms of
-jets of diffeomorphisms of 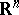 at zero (preserving the origin) acts. A vector field
at zero (preserving the origin) acts. A vector field  on
on 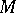 is called an infinitesimal automorphism, or a Killing field of the object
is called an infinitesimal automorphism, or a Killing field of the object 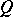 , if the corresponding (local) one-parameter group of transformations
, if the corresponding (local) one-parameter group of transformations 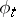 of
of 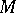 induces a group
induces a group 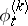 of transformations of the manifold of frames
of transformations of the manifold of frames 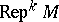 preserving
preserving 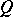 :
: 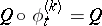 . The equation determining a Killing field of the object
. The equation determining a Killing field of the object 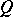 is called a Lie–Killing equation and the operator corresponding to it is called the Lie operator [6].
is called a Lie–Killing equation and the operator corresponding to it is called the Lie operator [6].
References
| [1] | W. Killing, "Ueber die Grundlagen der Geometrie" J. Reine Angew. Math. , 109 (1892) pp. 121–186 |
| [2] | P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian) |
| [3] | L.P. Eisenhart, "Riemannian geometry" , Princeton Univ. Press (1949) |
| [4] | S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) |
| [5] | S. Kobayashi, "Transformation groups in differential geometry" , Springer (1972) |
| [6] | A. Kumpera, D. Spencer, "Lie equations" , 1. General theory , Princeton Univ. Press (1972) |
| [7] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 2 , Interscience (1969) |
| [8] | I.P. Egorov, "Motions in spaces of affine connection" , Motions, spaces, affine connections , Kazan' (1965) pp. 5–179 (In Russian) |
Killing vector. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Killing_vector&oldid=47499