Killing space
From Encyclopedia of Mathematics
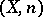
A fibre space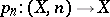 
for which the homotopy groups 
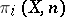vanish if 
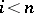, and 
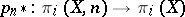is an isomorphism if 
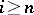. The space 
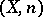is constructed by induction with respect to 
. If 
has already been constructed, then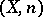 
is taken to be the homotopy fibre of the canonical mapping
 |
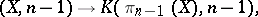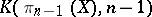denoting an Eilenberg–MacLane space. The sequence of spaces 
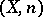and mappings 
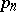is a Moore–Postnikov system of the mapping 
.
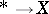Comments
See also [a1], Chapt. 8, Sect. 3.
References
| [a1] | E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966) pp. Chapt. 2 |
How to Cite This Entry:
Killing space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Killing_space&oldid=47498
Killing space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Killing_space&oldid=47498
This article was adapted from an original article by A.F. Kharshiladze (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article