Kernel pair

of a morphism in a category

A categorical generalization of the equivalence relation induced by a mapping of one set into another. A pair of morphisms 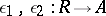in a category 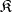is called a kernel pair of the morphism 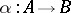if , and if for any pair of morphisms 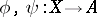for which there is a unique morphism 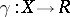such that 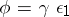and .

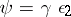Let 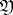be an arbitrary category of universal algebras of a given type and all homomorphisms between them that is closed with respect to finite products, and let 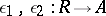be a kernel pair of a homomorphism 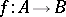in 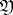. Then the image of the homomorphism

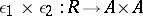induced by the pair 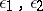is a congruence on the algebra 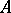(cf. also Congruence (in algebra)). Conversely, if 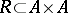is an arbitrary congruence on 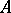, 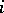is the imbedding of 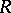into 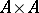, and 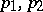are the projections of 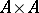onto 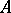, then the pair of homomorphisms 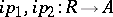is a kernel pair of the natural homomorphism of 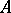onto the quotient algebra .

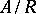In an arbitrary category with finite products and kernels of pairs of morphisms (cf. Kernel of a morphism in a category), the kernel pair of a morphism 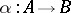is constructed as follows. One chooses a product with the projections 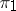and , and determines the kernel 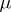of the pair of morphisms 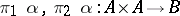. Then the pair of morphisms 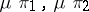is a kernel pair of .

Comments

A cokernel pair is defined dually.

References

[a1]	F.G. Manes, "Algebraic categories" , Springer (1976) pp. Chapt. 2, §1
[a2]	H. Schubert, "Kategorien" , 2 , Springer (1970) pp. Sect. 18.4
[a3]	S. MacLane, "Categories for the working mathematician" , Springer (1971) pp. Sects. 3.3, 3.4