Julia set

G. Julia [a1] and P. Fatou

studied the iteration of rational mappings . Let denote the -fold composite of the function with itself. A point is an element of the so-called Fatou set of if there exists a neighbourhood of in such that the family of iterates 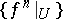is a normal family. The Julia set 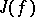is the complement of the Fatou set. 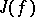has the following properties: 1) is non-empty and perfect (cf. Perfect set); 2) 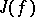equals the closure of the set of repelling periodic points (cf. Periodic point); 3) 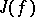is either totally disconnected (cf. Totally-disconnected space) or connected by Jordan arcs or coincides with ; 4) is invariant with respect to and 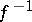; and 5) is an attractor (cf. Strange attractor) of the inverse iterated mapping 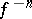. In almost-all cases 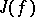has a fractal dimension and may be termed a fractal (cf. Fractals). D. Sullivan has given an exhaustive classification of the elements of the Fatou set with respect to their dynamics. Every component of is either periodic or pre-periodic. Let 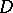be such a periodic domain and let be its period. Writing 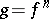one has the following five kinds of dynamics:

a) 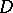is an attracting domain; 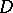contains an attracting periodic point 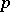with .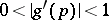

b) 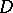is a super-attractive domain; contains a periodic point 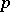which is also a critical point, i.e. .

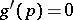c) 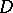is a parabolic domain; its boundary contains a periodic point with .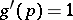

d) is a Siegel disc (cf. Siegel domain); 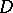is simply connected and is analytically equivalent to a rotation.

e) 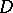is a Herman ring: 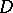is conformally equivalent to an annulus and 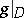is analytically conjugate to a rigid rotation of an annulus.

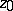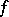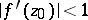Here a pre-periodic point is a point some iterate of which is periodic. A fixed point of is super-attractive if . (Recall that if is a fixed point, then is attractive if and repelling if .)

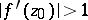The existence of Herman rings has been proved, but they have never yet (1989) been observed.

The best studied case is the quadratic mapping . All phenomena are present there, with the exception of a Herman ring. All for which is connected form the Mandelbrot set, the bifurcation diagram of 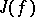in the parameter space of . See also Chaos; Routes to chaos.

References

[a1]	G. Julia, "Mémoire sur l'iteration des fonctions rationnelles" J. de Math. , 8 (1918) pp. 47–245
[a2a]	P. Fatou, "Sur les équations fonctionnelles" Bull. Soc. Math. France , 47 (1919) pp. 161–271
[a2b]	P. Fatou, "Sur les équations fonctionnelles" Bull. Soc. Math. France , 48 (1920) pp. 33–94
[a2c]	P. Fatou, "Sur les équations fonctionnelles" Bull. Soc. Math. France , 48 (1920) pp. 208–314
[a3]	H. Brolin, "Invariant sets under iteration of rational functions" Ark. Mat. , 6 (1965) pp. 103–144
[a4]	P. Blanchard, "Complex analytic dynamics" Bull. Amer. Math. Soc. , 11 (1984) pp. 84–141
[a5]	R.L. Devaney, "An introduction to chaotic dynamical systems" , Benjamin/Cummings (1986)
[a6]	H.-O. Peitgen, P.H. Richter, "The beauty of fractals" , Springer (1986)