Involutive distribution

The geometric interpretation of a completely-integrable differential system on an -dimensional differentiable manifold of class , . A -dimensional distribution (or a differential system of dimension ) of class , , on is a function associating to each point a -dimensional linear subspace of the tangent space such that has a neighbourhood with vector fields on it for which the vectors form a basis of the space at each point . 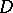The distribution is said to be involutive if for all points ,

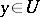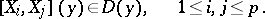This condition can also be stated in terms of differential forms. The distribution is characterized by the fact that

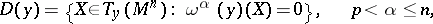where are -forms of class , linearly independent at each point ; in other words, is locally equivalent to the system of differential equations 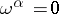. Then is an involutive distribution if there exist -forms on 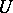such that

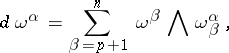that is, the exterior differentials 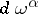belong to the ideal generated by the forms .

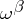A distribution of class on is involutive if and only if (as a differential system) it is an integrable system (Frobenius' theorem).

References

[1]	C. Chevalley, "Theory of Lie groups" , 1 , Princeton Univ. Press (1946)
[2]	R. Narasimhan, "Analysis on real and complex manifolds" , North-Holland & Masson (1968) (Translated from French)