Invariant test
A statistical test based on an invariant statistic. Let 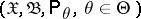 be a sampling space and suppose that the hypothesis
be a sampling space and suppose that the hypothesis 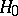 :
: 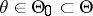 is tested against the alternative
is tested against the alternative 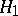 :
: 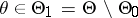 , where the hypothesis
, where the hypothesis 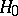 is invariant under the group
is invariant under the group 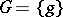 of one-to-one
of one-to-one 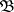 -measurable transformations of the space
-measurable transformations of the space 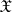 onto itself, that is,
onto itself, that is,
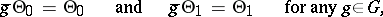 |
where 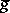 is the element of the group
is the element of the group 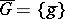 of one-to-one transformations of the probability measures
of one-to-one transformations of the probability measures 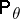 :
: 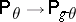 , defined for all
, defined for all 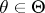 and
and 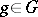 according to the formula
according to the formula 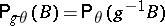 ,
, 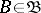 . Since
. Since 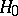 is invariant under
is invariant under 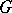 , in testing
, in testing 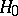 it is natural to use a test based on an invariant statistic with respect to this same group
it is natural to use a test based on an invariant statistic with respect to this same group  . Such a test is called an invariant test, and the class of all invariant tests is the same as the class of tests based on a maximal invariant. In the theory of invariant tests, the Hunt–Stein theorem plays an important role: If the hypothesis
. Such a test is called an invariant test, and the class of all invariant tests is the same as the class of tests based on a maximal invariant. In the theory of invariant tests, the Hunt–Stein theorem plays an important role: If the hypothesis  is invariant under the group
is invariant under the group  , then there exists a maximin test in the class of invariant tests for testing
, then there exists a maximin test in the class of invariant tests for testing  . An invariant test is a special case of an invariant statistical procedure (see Invariance of a statistical procedure).
. An invariant test is a special case of an invariant statistical procedure (see Invariance of a statistical procedure).
References
| [1] | E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1988) |
| [2] | L. Schmetterer, "Introduction to mathematical statistics" , Springer (1974) (Translated from German) |
| [3] | W.J. Hall, R.A. Wijsman, J.K. Chosh, "The relationships between sufficiency and invariance with applications in sequential analysis" Ann. Math. Stat. , 36 (1965) pp. 575 |
| [4] | G.P. Klimov, "Invariant inferences in statistics" , Moscow (1973) (In Russian) |
| [5] | S. Zacks, "The theory of statistical inference" , Wiley (1971) |
Invariant test. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Invariant_test&oldid=47420