Intersection index (in homology)

A homology invariant characterizing the algebraic (i.e. including orientation) number of points in the intersection of two subsets of complementary dimensions (in general position) in a Euclidean space or in an oriented manifold. In the case of a non-oriented manifold, the coefficient ring 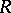for the homology is taken to be .

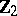Let 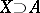, 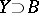be pairs of subsets in the Euclidean space 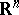such that , and let be the mapping given by 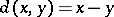. The intersection index 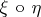for the homology classes 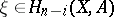, is the element . Here 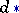is the induced homology mapping, while 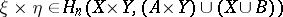is the exterior homology product of the elements 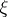and .

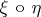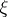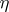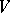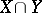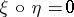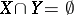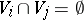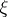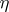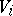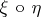The intersection index depends only on those parts of the classes and with supports in an arbitrary small neighbourhood of the closure of the set . In particular, if . Also, if , for , then the local intersection indices of and corresponding to each open set are defined, and their sum coincides with . The invariant does not change under homeomorphisms of . In conjunction with the previous property of locality, this enables one to determine the intersection index for compact subsets of an oriented variety. The following anti-commutative relation holds:

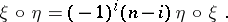If 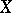and 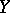are vector subspaces in general position, if 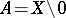, , and if 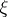and 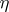are generators of 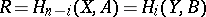, then 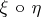is a generator of 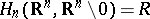. Since the choice of these generators is equivalent to the choice of an orientation in the corresponding Euclidean spaces, this makes it possible to determine the intersection index 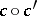for two chains of complementary dimensions (including singular ones) for which 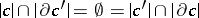( 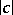is the support of the chain , the boundary of which is 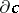). Then 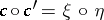for certain chains and of the homology classes , , , , , .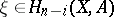

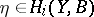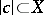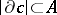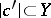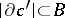The intersection index is used to describe certain duality relations in manifolds.

References

[1]	A. Dold, "Lectures on algebraic topology" , Springer (1980)

References

[a1]	J.F. Adams, "Stable homotopy and generalised homology" , Univ. Chicago Press (1974)