Incomplete gamma-function

The function defined by the formula

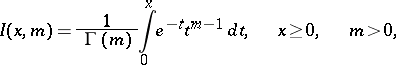where is the gamma-function. If 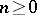is an integer, then

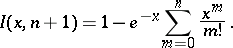Series representation:

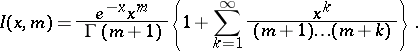Continued fraction representation:

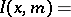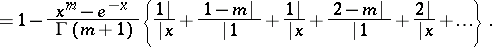Asymptotic representation for large :

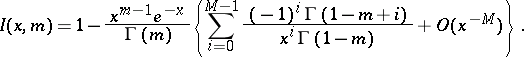Asymptotic representation for large :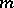

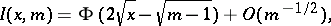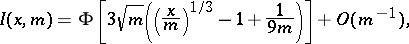where

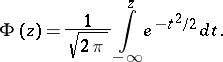Connection with the confluent hypergeometric function:

Connection with the Laguerre polynomials :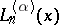

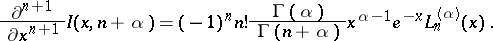Recurrence relation:

References

[1]	M. Abramowitz, I.A. Stegun, "Handbook of mathematical functions" , Dover, reprint (1973)
[2]	V.I. Pagurova, "Tables of the incomplete gamma-function" , Moscow (1963) (In Russian)

Comments

The following notations are also used:

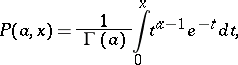with 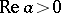, . The -function is related to the confluent hypergeometric function:

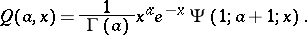New asymptotic expansions for both and 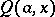are given in [a1].

References

[a1]	N.M. Temme, "The asymptotic expansion of the incomplete gamma functions" SIAM J. Math. Anal. , 10 (1979) pp. 757–766