Homology functor

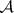A functor on an Abelian category that defines a certain homological structure on it. A system of covariant additive functors from an Abelian category into an Abelian category is called a homology functor if the following axioms are satisfied.

1) For each exact sequence

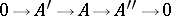and each , in a morphism is given, which is known as the connecting or boundary morphism.

2) The sequence

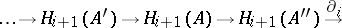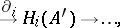called the homology sequence, is exact.

Thus, let be the category of chain complexes of Abelian groups, and let 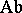be the category of Abelian groups. The functors 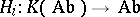which assign to a complex 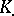the corresponding homology groups 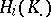define a homology functor.

Let 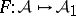be an additive covariant functor for which the left derived functors (, 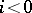) are defined (cf. Derived functor). The system will then define a homology functor from 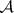into .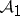

Another example of a homology functor is the hyperhomology functor.

A cohomology functor is defined in a dual manner.

References

[1]	A. Grothendieck, "Sur quelques points d'algèbre homologique" Tohoku Math. J. , 9 (1957) pp. 119–221