Holomorphically-convex complex space

A complex space that satisfies the following condition: For each compactum the set

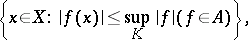where is the algebra of holomorphic functions on , is compact. A space is holomorphically convex if and only if it admits a proper surjective holomorphic mapping onto some Stein space (a holomorphically-complete space) which induces an isomorphism between the algebras of holomorphic functions on these spaces. The mapping (the holomorphic reduction of ) is uniquely defined and has connected fibres [1]. For any coherent analytic sheaf 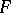on a holomorphically-convex complex space , the cohomology spaces 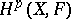and 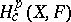, , 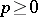are separable topological vector spaces [2].

A special class of holomorphically-convex complex spaces is formed by the complex spaces 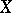for which the holomorphic reduction mapping is bijective outside some compact analytic set (such a space is obtained from a Stein space by a proper modification which blows-up a finite number of points). A complex space possesses this property if and only if

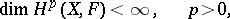for any coherent analytic sheaf on 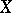[3]. This class of complex spaces also coincides with the class of strictly 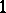-convex complex spaces (cf. Pseudo-convex and pseudo-concave).

References

[1]	H. Cartan, "Quotients of complex analytic spaces" , Contributions to function theory. Internat. Colloq. Function Theory, Bombay 1960 , Tata Inst. (1960) pp. 1–15
[2]	J.P. Ramis, "Théorèmes de séperation et de finitude pour l'homologie et la cohomologie des espaces 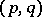-convexes-concaves" Ann. Scuola Norm. Sup. Pisa Ser. 3 , 27 (1973) pp. 933–997
[3]	R. Narasimhan, "The Levi problem for complex spaces II" Math. Ann. , 146 (1962) pp. 195–216

References

[a1]	R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Sect. G
[a2]	H. Grauert, R. Remmert, "Theory of Stein spaces" , Springer (1979) (Translated from German)
[a3]	H. Grauert, R. Remmert, "Komplexe Räume" Math. Ann. , 136 (1958) pp. 245–318