Gårding inequality

An inequality of the form

where is a complex-valued function with compact support (in 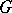), is a bounded domain and

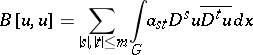is a quadratic integral form with complex continuous coefficients 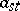in 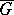. A sufficient condition for the Gårding inequality to be valid for any function 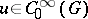is the existence of a positive constant such that

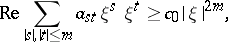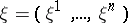for any and all real vectors . Formulated and proved by L. Gårding [1].

References

[1]	L. Gårding, "Dirichlet's problem for linear elliptic partial differential equations" Math. Scand. , 1 (1953) pp. 55–72
[2]	K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, §1

Comments

A sharp form of this inequality has been given by L. Hörmander. See [a1], Sects. 18.1, 18.6, and the literature quoted there.

References

[a1]	L.V. Hörmander, "The analysis of linear partial differential operators" , 3 , Springer (1985)